Unit 1: Working with Decimals
Topic A: Part of the Whole Thing
This is the beginning of an adventure with numbers that represent part of the whole thing. These numbers can be shown in a few different ways:
| Fraction name | Example |
|---|---|
| Decimal fraction | 0.50 |
| Common fraction | [latex]\dfrac{1}{2}[/latex] or [latex]\dfrac{50}{100}[/latex] |
| Percent fraction | 50% |
When we talk about fractions in any of the three ways listed above, we are talking about numbers in relation to the whole thing. What do we mean by “the whole thing”? We mean one complete thing.
An example would be one full jug of juice. That is 1 whole thing.

Once someone starts taking some juice, less than the whole thing remains. Now, half of the juice is gone.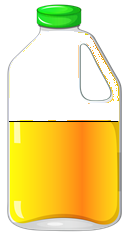 The remaining amount can be written as
The remaining amount can be written as
- half of one whole thing
- 0.5
- ½
- 50%
Now almost all the juice has been taken.
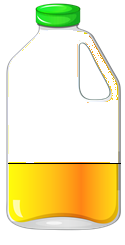 The remaining amount can be written as
The remaining amount can be written as
- 0.25
- ¼
- 25%
Now there are two full jugs of juice. This shows two whole things.

A fraction does not tell us much unless we know what the fraction is part of—we need to know exactly what the whole thing is! If someone says to you, “Sure, let’s go! I still have half!” you really need to know, “Half of what?”
The answer could be [latex]\frac{1}{2}[/latex] a tank of gas, or [latex]\frac{1}{2}[/latex] a paycheque, or [latex]\frac{1}{2}[/latex] a vacation, or [latex]\frac{1}{2}[/latex] an hour.
Decimal Fractions
Decimal fractions are one way to consider parts of the whole thing. The whole thing = 1.
You use decimal fractions every time you think about money. The dollars are written as whole numbers and the cents are written as a decimal fraction of a dollar.
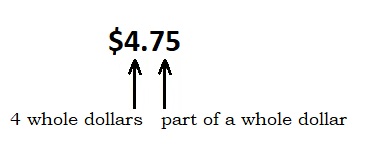 A decimal fraction has a decimal point that separates the whole number from the fraction. The decimal point looks like this:
A decimal fraction has a decimal point that separates the whole number from the fraction. The decimal point looks like this: ![]()
A whole pizza might be divided into eight pieces, or ten pieces, or twelve pieces. However, for decimal fractions the whole is always divided into ten pieces, which are called tenths. This is because we use a decimal system based on the number ten (“deci” comes from “decimus,” the Latin word for “tenth”). The tenths are also divided into ten pieces to make hundredths. And then the hundredths are divided by ten to make thousandths, and so on.
Decimal fractions are often used in our daily lives, especially for money and measurement. For example:
- The total was $12.24.
- It is 3.5 kilometres from my house to the store.
- It costs $1.99 per kilogram for apples.
- She caught a 4.8 kilogram salmon.
You will be working with decimal fractions in the first two units of this book.


Common Fractions
Common fractions are a second way we will work with parts of the whole thing.
They are written with two numbers, one above the other, with a line in between. The line may be straight, or it may be on an angle.
[latex]\frac{3}{4}[/latex] or ¾
The numerator is the top number in a common fraction. The numerator tells how many parts. The denominator is the bottom number. The denominator tells how many equal parts there are in the whole thing.
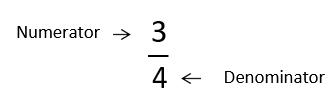
Example A
 The whole thing is 1 pizza. This pizza has been cut into 8 equal pieces. The denominator is 8.
The whole thing is 1 pizza. This pizza has been cut into 8 equal pieces. The denominator is 8.
As a fraction, the whole thing is [latex]\tfrac{8}{8}[/latex] (eight-eigths).
If I ate 3 pieces, that would be [latex]\tfrac{3}{8}[/latex] of the pizza.
Here are some things to keep in mind while you complete the following exercise:
- [latex]\text{one quarter}=0.25=\frac{1}{4}[/latex]
- [latex]\text{one third}= 0.333.. =\frac{1}{3}[/latex]
- [latex]\text{one half}=0.5=\frac{1}{2}[/latex]
- [latex]\text{two thirds}= 0.666...=\frac{2}{3}[/latex]
- [latex]\text{three quarters}=0.75=\frac{3}{4}[/latex]
Exercise 1
Look at the pictures and use a fraction to answer the questions.
- How much gas is left?
- Do you want more coffee?
-

No thanks, I still have ______ of a cup. -

Sure, I only have ______ a cup. -

Yes please, I’m down to _______ of a cup.
-
- Do we need more juice?
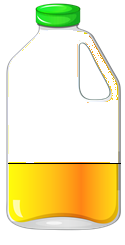
Yes, there is just _____ of the juice.
Answers to Exercise 1
Answers may differ because the fraction is approximate. Ask your instructor to check any different answers.
- Gas
- [latex]\tfrac{1}{2}[/latex] or [latex]\frac{2}{4}[/latex] or 0.5
- [latex]\tfrac{1}{4}[/latex] or 0.25
- Coffee
- [latex]\tfrac{3}{4}[/latex] or 0.75
- [latex]\tfrac{1}{2}[/latex] or 0.5
- [latex]\tfrac{1}{4}[/latex]
- Juice – [latex]\tfrac{1}{3}[/latex]
Fractions as a Percent
A third and useful way to think about parts of the whole thing is as a percent.
Percent fractions are written with a number and a percent sign.
1% 12% 50% 99%
In percent fractions, there is always a denominator of 100. That makes the arithmetic much easier and helps us to understand the fraction.
For example, if you got [latex]\tfrac{14}{20}[/latex] on a test last week, and [latex]\tfrac{13}{17}[/latex] on a test this week, it is hard to get a sense of how you are doing. But if you know you got 70% last week and 76% this week, it is easier to see your improvement.
In percent fractions, the whole thing is 100% so 100% equals 1.
Statistics and general information are often reported in percent fractions.


What is a Decimal Fraction?
As you know, fractions describe part of the whole thing—a fraction is smaller than 1. And as you also know, 1 (the whole thing) can be many things. For example, it can be:
- 1 dollar
- 1 city
- 1 school
- 1 pay cheque
- 1 year
- 1 second
- 1 loaf of bread
- 1 ferry ride
A decimal might represent part of a year, part of the population of Canada, part of an hour, or part of anything.
Decimal fractions are different from common fractions in several ways:
- A decimal point separates whole numbers from the fraction.
[latex]\dfrac{1}{10} = 0.1[/latex] [latex]\dfrac{34}{100} = 0.34[/latex] [latex]\dfrac{5}{10} = 0.5[/latex] - In a decimal fraction, there is no denominator.
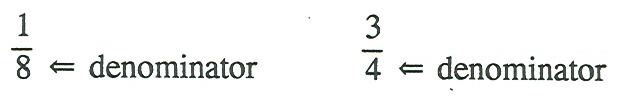
We tell the size of the denominator by looking at how many numerals are placed after the decimal point.
Decimal fraction denominators are always ten or ten multiplied by tens. Decimal means “based on the number ten”.
[latex]\large\begin{array}{ll} 0.4&\text{has a denominator of 10}\\ 0.44&\text{has a denominator of 100}\\ 0.444&\text{has a denominator of 1 000}\\ 0.4444&\text{has a denominator of 10 000}\\ 0.44444&\text{has a denominator of 100 000}\\ 0.444444&\text{has a denominator of 1 000 000}\\ \end{array}[/latex]
A whole number and a decimal can be written together. This is called a mixed decimal.
4.35 100.47 $12.39
Every whole number has a decimal point after it, even though we usually do not bother to write the decimal point unless apart of the whole (fraction) follows the whole number.
We can also put zeros to the right of the decimal point of any whole number without changing its value. Get used to thinking of a decimal point after every whole number!
[latex]\begin{array}{ccrcl} 3&=&3.&=&3.0000000\\ 275&=&275.&=&275.0\\ 100&=&100.&=&100.0000000000\\ $8&=&$8.&=&$8.00\end{array}[/latex]
e.g., ⅔, ³⁄₇ , ⁴⁹⁄₅₀
The top number in a common fraction; the numerator tells how many parts of the whole thing are being considered.
The bottom number in a common fraction; tells into how many equal parts the whole thing has been divided.
For every one hundred.
A whole number and a decimal fraction. 1.75



