Unit 3: Multiplying & Dividing Fractions
Topic A: Multiplying Fractions
Example A
What is [latex]\tfrac{1}{4}[/latex] of 4? (HINT: Replace the word of with a × sign)
Here are four equal shapes:
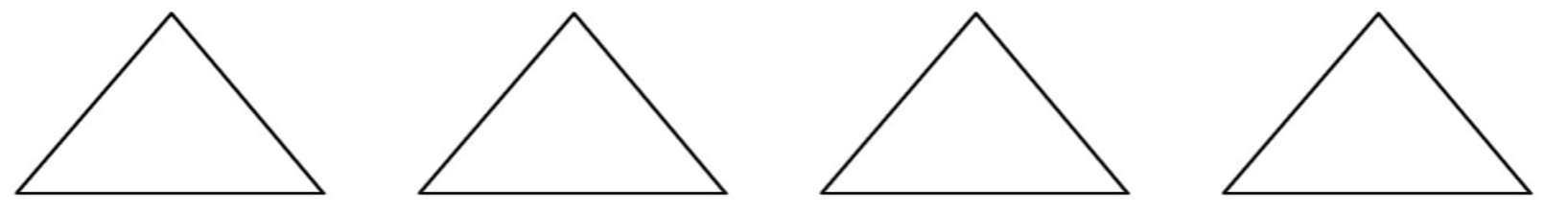
Shade in [latex]\tfrac{1}{4}[/latex] of the shapes.
You should have one shape shaded.
You have just done this multiplication question:
[latex]\dfrac{1}{4}\times4=1[/latex] or [latex]\dfrac{1}{4}\text{ of }4=1[/latex]
Example B
What is [latex]\tfrac{2}{5}[/latex] of 5?
Draw 5 equal shapes:
Shade in [latex]\tfrac{2}{5}[/latex] of the shapes.
You should have shaded in two shapes.
[latex]\tfrac{2}{5}\times5=2[/latex] or [latex]\tfrac{2}{5}\text{ of }5=2[/latex]
Example C
What is [latex]\tfrac{1}{2}[/latex] of 10?
Here are 10 equal shapes:

Shade in [latex]\tfrac{1}{2}[/latex] of the shapes.
Did you shade 5?
[latex]\dfrac{1}{2}\times10=5[/latex] or [latex]\dfrac{1}{2}\text{ of }10=5[/latex]
Example D
What is [latex]\tfrac{1}{4}[/latex] of 8?

Shade in [latex]\tfrac{1}{4}[/latex] of the shapes.
First, divide the 8 shapes into 4 equal groups.
Now shade 1 group. [latex]\dfrac{1}{4}\times8=2[/latex] or [latex]\dfrac{1}{4}\text{ of }8=2[/latex]
Example E
What is [latex]\tfrac{2}{3}[/latex] of 6?
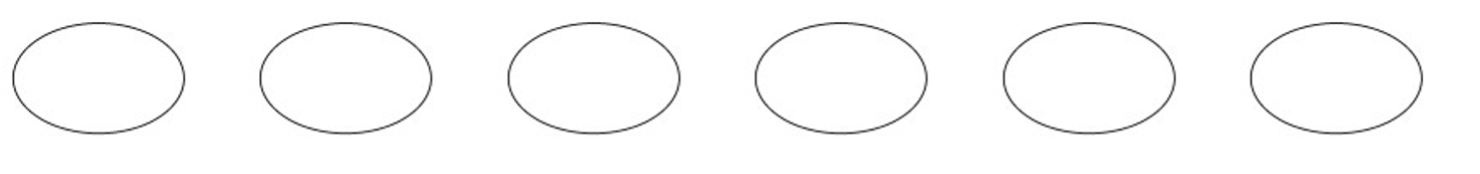
Shade in [latex]\tfrac{2}{3}[/latex] of the shapes.
First, divide the 6 shapes into 3 equal groups.
Now shade 2 group. [latex]\dfrac{2}{3}\times6=4[/latex]
These examples calculate a fraction of a whole number. Some everyday examples, with the answers, are listed:
- I burned [latex]\tfrac{1}{2}[/latex] of the hamburger patties. There were 8 patties. How many patties were burned? [latex]\tfrac{1}{2}[/latex] of 8 patties = [latex]\tfrac{1}{2}\times8=4[/latex] burned patties.
- Mary only finished [latex]\tfrac{3}{4}[/latex] of the test. The test had 20 questions. How many questions did Mary do? [latex]\tfrac{3}{4}[/latex] of test = [latex]\tfrac{3}{4}[/latex] of 20 questions = [latex]\tfrac{3}{4}\times20=15[/latex] questions.
- [latex]\tfrac{1}{5}[/latex] of the employees have been laid off. There are 50 employees. How many have been laid off? [latex]\tfrac{1}{5}[/latex] of 50 employees = [latex]\tfrac{1}{5}\times50=10[/latex] employees laid off.
- We spend [latex]\tfrac{1}{4}[/latex] of our monthly take-home pay on rent. Our take-home pay is $1600. How much is the rent? [latex]\tfrac{1}{4}[/latex] of pay = [latex]\tfrac{1}{4}[/latex] of $1600 = [latex]\tfrac{1}{4}\times\$1600=\$400[/latex] on rent
Exercise 1
Write the multiplication equation you would use to find the fraction of the whole number. You do not have to calculate the answers.
- More than [latex]\tfrac{1}{3}[/latex] of the students are single parents. There are 27 students. How many students are single parents?
- We have ten houses on our street [latex]\tfrac{2}{5}[/latex] of the houses have cedar shake roofs. How many houses have cedar shake roofs?
- The guinea hen hatched 16 chicks. The ravens snatched [latex]\tfrac{3}{8}[/latex] of the chicks. How many chicks did the ravens take?
Answers to Exercise 1
- [latex]\dfrac{1}{3}\times27=9[/latex]
- [latex]\dfrac{2}{5}\times10=4[/latex]
- [latex]\dfrac{3}{8}\times16=6[/latex]
Now let’s look at multiplying a fraction by a whole number:
- [latex]4\times\dfrac{1}{3}=[/latex]
- [latex]3\times\dfrac{4}{5}=[/latex]
- [latex]22\times\dfrac{1}{4}=[/latex]
The order of writing the multiplication equation will not change the product, but it does change how we understand what the numbers mean. Again, look at the examples:
Example F
[latex]4\times\tfrac{1}{2}[/latex] means you have four halves.
Imagine tomatoes cut in half and you have 4 halves.
How many tomatoes would you have altogether?

4 halves = 2 tomatoes
[latex]4\times\dfrac{1}{2}=2[/latex]
Example G
[latex]3\times\tfrac{1}{4}[/latex] means that you have [latex]\tfrac{1}{4}[/latex] of something three times. Imagine that you spent [latex]\tfrac{1}{4}[/latex] of an hour exercising in the morning, [latex]\tfrac{1}{4}[/latex] of an hour exercising after lunch, and [latex]\tfrac{1}{4}[/latex] of an hour exercising in the evening.
How long did you exercise?
[latex]3\times\dfrac{1}{4}[/latex] hour = [latex]\dfrac{3}{4}[/latex] hour = three quarters of an hour
Here are some everyday examples of multiplying a fraction by a whole number:
- There are six boxes of cereal open in the cupboard, and each one is ⅓ full.
It is the same as having full boxes of cereal.- [latex]6\times\tfrac{1}{3}\text{ box of cereal} =\tfrac{6}{3} = 2 \text{ boxes of cereal}[/latex]
- We have three packs of ground beef that are [latex]\tfrac{1}{2}[/latex] full. How much meat is there altogether?
- [latex]3\times\tfrac{1}{2} \text{ pack of meat }= \tfrac{3}{2} = 1\tfrac{1}{2}\text{ packs of meat}[/latex]
- How much gas do we have for the motorboat? There are 4 jerry cans (cans for carrying gas), each about [latex]\tfrac{1}{4}[/latex] full.
- [latex]4\times\tfrac{1}{4}\text{ cans of gas}= \tfrac{4}{4} = 1\text{ can of gas}[/latex]
Exercise 2
Write the multiplication equation you would use to multiply a fraction by a whole number. You do not have to calculate the
answer.
- I should buy more shampoo, but this bathroom has five bottles of the stuff lying around! Each bottle is about [latex]\frac{1}{8}[/latex] full. How much shampoo is there altogether?
- When we double a recipe, we multiply each ingredient by 2. Double a recipe that uses [latex]\frac{1}{4}[/latex] teaspoon of nutmeg. How much nutmeg is needed?
Answers to Exercise 2
- [latex]5\times\dfrac{1}{8}=\dfrac{3}{4}[/latex]
- [latex]\dfrac{1}{4}\times2=\dfrac{1}{2}[/latex]
Multiplying a Whole Number and a Proper Fraction
Any whole number can be written with a denominator of 1. (This does not change the value of the whole number because a number divided by one is still the same whole number in the end.)
- [latex]1=\dfrac{1}{1}[/latex]
- [latex]2=\dfrac{2}{1}[/latex]
- [latex]2=\dfrac{3}{1}[/latex]
- [latex]4=\dfrac{4}{1}[/latex]
- [latex]100 = \dfrac{100}{1}[/latex]
- and so on.
To multiply a whole number and a fraction, do this:
Step 1: Write the whole number as a fraction with a denominator of 1.
Step 2: Multiply the numerator by the numerator.
Step 3: Multiply the denominator by the denominator.
Step 4: Simplify the product
Example H
[latex]\dfrac{4}{6}\times6=[/latex]
Step 1: Write the whole number with a denominator of 1.
[latex]\dfrac{4}{5}\times\dfrac{6}{1}=[/latex]
Step 2: Multiply the numerators.
[latex]\dfrac{4}{5}\times\dfrac{6}{1}=\dfrac{24}{}[/latex]
Step 3: Multiply the denominators.
[latex]\dfrac{4}{5}\times\dfrac{6}{1}=\dfrac{24}{5}[/latex]
Step 4: Write your answer in lowest terms.
Since [latex]\begin{array}{r}4\\ 5\enclose{longdiv}{24}\\20\\ \hline 04\end{array}[/latex] [latex]\dfrac{24}{5}=4\dfrac{4}{5}[/latex] Then [latex]\dfrac{4}{6}\times 6 = 4 \dfrac{4}{5}[/latex]
Example I
[latex]\dfrac{1}{2}\times 3=[/latex]
Step 1:
[latex]\dfrac{1}{2}\times\dfrac{3}{1}=[/latex]
Step 2 & 3:
[latex]\dfrac{1}{2}\times\dfrac{3}{1}[/latex] = [latex]\dfrac{3}{2}[/latex]
Step 4: Write your answer in lowest terms.
Since [latex]\begin{array}{r}1\\ 2\enclose{longdiv}{3}\\-2\\ \hline 1\end{array}[/latex] [latex]\dfrac{3}{2}[/latex] = [latex]1\dfrac{1}{2}[/latex] Then [latex]\dfrac{1}{2}[/latex] × 3= [latex]1\dfrac{1}{2}[/latex]
Example J
[latex]\dfrac{2}{3}\times4 =[/latex]
[latex]\dfrac{2}{3}\times\dfrac{4}{1}=\dfrac{8}{3}=2\dfrac{2}{3}[/latex]
[latex]\begin{array}{r}2\\ 3\enclose{longdiv}{8}\\-6\\ \hline 2\end{array}[/latex]
Example K
[latex]7 \times \dfrac{3}{4}[/latex] =
[latex]\dfrac{7}{1} \times \dfrac{3}{4} = \dfrac{21}{4} = 5\dfrac{1}{4}[/latex]
[latex]\begin{array}{r}5\\ 4\enclose{longdiv}{21}\\ −20\\ \hline 1\end{array}[/latex]
Exercise 3
Multiply these fractions. Write your answers in lowest terms.
- [latex]\begin{array}{l}\dfrac{3}{5}\times10= \\ \dfrac{3}{5}\times\dfrac{10}{1}=\dfrac{30}{5}=6 \end{array}[/latex]
[latex]\begin{array}{r}6\\ 5\enclose{longdiv}{30}\\ −30\\ \hline 0\end{array}[/latex] - [latex]\begin{array}{l} 8\times\dfrac{1}{10}=\\ \dfrac{8}{1}\times\dfrac{1}{10}=\dfrac{8\div2}{10\div2}=\dfrac{4}{5} \end{array}[/latex]
- [latex]\dfrac{2}{3}\times9=[/latex]
- [latex]4\times\dfrac{1}{6}=[/latex]
- [latex]1\times\dfrac{3}{8}=[/latex]
- [latex]\dfrac{1}{2}\times5=[/latex]
- [latex]5\times\dfrac{2}{3}=[/latex]
- [latex]\dfrac{1}{2}\times8=[/latex]
- [latex]6\times\dfrac{1}{5}=[/latex]
- [latex]\dfrac{3}{2}\times12=[/latex]
- [latex]\dfrac{3}{8}\text{ of }4 =[/latex]
- [latex]\dfrac{7}{8}\text{ of }3 =[/latex]
Answers to Exercise 3
- 6
- [latex]\dfrac{4}{5}[/latex]
- 6
- [latex]\dfrac{2}{3}[/latex]
- [latex]\dfrac{3}{8}[/latex]
- [latex]2\dfrac{1}{2}[/latex]
- [latex]3\dfrac{1}{3}[/latex]
- 4
- [latex]1\dfrac{1}{5}[/latex]
- 18
- [latex]1\dfrac{1}{2}[/latex]
- [latex]2\dfrac{5}{8}[/latex]
Multiplying Common Fractions Together
To multiply common fractions, multiply the numerator of the first fraction by the numerator of the second, and then multiply the denominator of the first fraction by the denominator of the second. Then, simplify the answer (meaning, write the answer in lowest terms).
The method is easy, but let’s take a look at what you’re doing.
Example L
[latex]\dfrac{1}{2}\times \dfrac{1}{2}= \dfrac{1}{2}\text{ of }\dfrac{1}{2}[/latex] =
Take an apple and cut it in half.

Now cut one of the halves in half. What fraction of the whole apple do you get?

You get [latex]\dfrac{1}{4}[/latex] of the apple. [latex]\dfrac{1}{2} \times \dfrac{1}{2} = \dfrac{1}{4}[/latex]
Example M
You borrowed 3⁄4 of a bag of cement from your neighbour. You used 1⁄3 of the cement and gave the bag back to him. How much of your neighbour’s bag of cement did you use?

[latex]\dfrac{3}{4}[/latex] of a bag
Use [latex]\dfrac{1}{3}[/latex] of this
[latex]\dfrac{1}{3}[/latex] of [latex]\dfrac{3}{4} = \dfrac{1}{4}[/latex] of the bag used
You used [latex]\dfrac{1}{4}[/latex] of a bag.
Example N
You are making a marinade to tenderize that cheap steak you bought. It calls for [latex]\tfrac{2}{3}[/latex] cup of beer. You only need [latex]\tfrac{1}{2}[/latex] of the amount the recipe makes and it would be a shame to waste the beer. How much beer is needed?

[latex]\dfrac{1}{2}[/latex] of [latex]\dfrac{2}{3}[/latex]
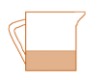 [latex]\dfrac{1}{3}[/latex] of a cup
[latex]\dfrac{1}{3}[/latex] of a cup
[latex]\dfrac{1}{2}[/latex] of [latex]\dfrac{2}{3}[/latex] cup of beer = [latex]\dfrac{1}{2}\times \dfrac{2}{3}[/latex] = [latex]\dfrac{2}{6}[/latex] = [latex]\dfrac{2}{6}[/latex] [latex]\dfrac{\div 2}{\div 2}[/latex] = [latex]\dfrac{1}{3}[/latex] cup of beer.
Exercise 4
Multiply these fractions. Write the answers in lowest terms
- [latex]\dfrac{1}{2}\times\dfrac{1}{2}[/latex] = [latex]\dfrac{1}{4}[/latex]
- [latex]\dfrac{1}{2}\times\dfrac{4}{2}[/latex] =
- [latex]\dfrac{3}{5}\times\dfrac{2}{4}[/latex] =
- [latex]\dfrac{2}{5}\times\dfrac{3}{5}[/latex] =
- [latex]\dfrac{5}{6}\times\dfrac{1}{4}[/latex] =
- [latex]\dfrac{5}{10}\times\dfrac{1}{4}[/latex] =
- [latex]\dfrac{1}{4}\times\dfrac{1}{8}[/latex] =
- [latex]\dfrac{8}{10}\times\dfrac{2}{3}[/latex] =
Answers to Exercise 4
- 1
- [latex]\dfrac{3}{10}[/latex]
- [latex]\dfrac{6}{25}[/latex]
- [latex]\dfrac{5}{24}[/latex]
- [latex]\dfrac{1}{8}[/latex]
- [latex]\dfrac{1}{32}[/latex]
- [latex]\dfrac{8}{15}[/latex]
Simplify Before Multiplying
Review Factors and Expressing Fractions in Lower Terms in Topic B. Multiplication of common fractions can be made much easier if you simplify before you multiply. In a multiplication question any numerator and any denominator may be divided by a common factor. This is sometimes called cancelling.
Step 1: Look to see if any numerator and any denominator have Common Factors. Choose the Greatest Common Factor.
Step 2: Divide that numerator and that denominator by the Greatest Common Factor (GCF ). Be sure to cross out the old numerals and put in the lower terms.
Step 3: Multiply the numerators (Be sure to use the lower term!) and then the denominators.
Step 4: Simplify.
Example O
[latex]\dfrac{3}{4}\times\dfrac{1}{6}[/latex]
Step 1: Numerator 3 and denominator 6 have a common factor of 3.
Step 2: [latex]\dfrac{\cancel{3}1}{4}\times\dfrac{1}{\cancel{6}2}[/latex] [latex]3\div3 = 1 \text{ and } 6 \div 3 = 2[/latex]
Step 3: [latex]\dfrac{\cancel{3}1}{4}\times\dfrac{1}{\cancel{6}2} = \dfrac{1}{8}[/latex]
Step 4: The answer is already in lowest terms.
Example P
[latex]\dfrac{3}{4}\times\dfrac{8}{9}=[/latex]
Step 1: Numerator 3 and denominator 9 have a common factor of 3, AND numerator 8 and denominator 4 have common factors of 2 and 4. The G.C.F. is 4.
Step 2: [latex]\dfrac{\cancel{3}1}{\cancel{4}1}\times\dfrac{\cancel{8}2}{\cancel{9}3}[/latex] 3 ÷ 3 = 1, 9 ÷ 3 = 3, 8 ÷ 4 = 2, and 4 ÷ 4 = 1
Step 3: = [latex]\dfrac{\cancel{3}1}{\cancel{4}1}\times\dfrac{\cancel{8}2}{\cancel{9}3}[/latex] = [latex]\dfrac{2}{3}[/latex]
Step 4: The answer is already in lowest terms.
Example Q
[latex]\dfrac{3}{8}\times12 =[/latex]
[latex]\dfrac{3}{8}\times\dfrac{12}{1}[/latex] (Numerator 12 and denominator 8 have a G.C.F. of 4)
[latex]\dfrac{3}{\cancel{8}2}\times\dfrac{\cancel{12}3}{1}=\dfrac{9}{2}=4\dfrac{1}{2}[/latex]
It is easier to simplify (or cancel) before you multiply because the numbers are smaller and the factors easier to find.
You may make fewer multiplying mistakes, too.
Exercise 5
Find the products. Simplify before multiplying when possible.
- [latex]\dfrac{5}{6}\times\dfrac{4}{5}=\dfrac{\cancel{5}1}{\cancel{6}3} \times \dfrac{\cancel{4}2}{\cancel{5}1} = \dfrac{2}{3}[/latex]
- [latex]\dfrac{3}{5}\times\dfrac{5}{9} =\dfrac{\cancel{3}1}{\cancel{5}1} \times \dfrac{\cancel{5}1}{\cancel{9}3} = \dfrac{1}{3}[/latex]
- [latex]\dfrac{3}{16}\times\dfrac{8}{9}[/latex] =
- [latex]\dfrac{2}{5}\times\dfrac{5}{8}[/latex] =
- [latex]\dfrac{4}{21}\times\dfrac{7}{8}[/latex] =
- [latex]\dfrac{9}{10}\times\dfrac{2}{3}[/latex] =
- [latex]\dfrac{2}{5}\times\dfrac{10}{7}[/latex] =
- [latex]\dfrac{3}{4}\times\dfrac{1}{12}[/latex] =
A multiplication question may have more than two fractions to be multiplied, such as
[latex]\dfrac{2}{3}\times\dfrac{9}{10}\times\dfrac{5}{8}=\dfrac{3}{8}[/latex]
Cancel any numerator with any denominator and then multiply all numerators together and then all denominators together. Study this worked example:
Numerator 2 and denominator 8 have a common factor of 2, numerator 9 and denominator 3 have a common factor of 3, AND numerator 5 and denominator 10 have a common factor of 5:
[latex]\dfrac{\cancel{2}1}{\cancel{3}1} \times \dfrac{\cancel{9}3}{\cancel{10}2} \times \dfrac{\cancel{5}1}{\cancel{8}4} = \dfrac{3}{8}[/latex]
- [latex]\dfrac{8}{9}\times\dfrac{3}{4} =[/latex]
- [latex]\dfrac{1}{2}\times\dfrac{2}{5} \times \dfrac{5}{7} =[/latex]
- [latex]\dfrac{3}{5}\times\dfrac{2}{3} \times \dfrac{1}{2} =[/latex]
- 2 × [latex]\dfrac{3}{4}\times\dfrac{5}{12} =[/latex]
- [latex]\dfrac{4}{9}\times\dfrac{3}{5}\times \dfrac{15}{16} =[/latex]
- [latex]\dfrac{2}{3}\times\dfrac{3}{4} \times \dfrac{8}{9} =[/latex]
Answers to Exercise 5
- [latex]\dfrac{1}{6}[/latex]
- [latex]\dfrac{1}{4}[/latex]
- [latex]\dfrac{1}{6}[/latex]
- [latex]\dfrac{3}{5}[/latex]
- [latex]\dfrac{4}{7}[/latex]
- [latex]\dfrac{1}{16}[/latex]
- [latex]\dfrac{2}{3}[/latex]
- [latex]\dfrac{1}{7}[/latex]
- [latex]\dfrac{1}{5}[/latex]
- [latex]\dfrac{5}{8}[/latex]
- [latex]\dfrac{1}{4}[/latex]
- [latex]\dfrac{4}{9}[/latex]
Multiplying Mixed Numbers
Review Renaming Mixed Numbers as Improper Fractions in Unit 1. To multiply with a mixed number, follow these steps:
Step 1: Rename any mixed numbers as improper fractions.
Step 2: Write any whole number by itself as an improper fraction with a denominator of 1.
Step 3: Rewrite the question with the new improper fraction(s).
Step 4: Simplify (cancel).
Step 5: Multiply the numerator by the numerator.
Multiply the denominator by the denominator.
Step 6: The answer will often be an improper fraction. Rename improper fractions as mixed numbers, and be sure any fraction is in lowest terms.
Example R
[latex]2\dfrac{3}{4} \times \dfrac{1}{3} =[/latex]
Step 1: [latex]2\dfrac{3}{4} = \dfrac{11}{4}[/latex]
Step 2: No whole numbers by themselves.
Step 3: Question is rewritten as [latex]\dfrac{11}{4}\times \dfrac{1}{3}[/latex]
Step 4: Simplify – the fraction has no common factors. Can’t simplify.
Step 5: [latex]\dfrac{11}{4} \times \dfrac{1}{3} = \dfrac{11}{12}[/latex]
Step 6: Already in lowest terms.
Example S
[latex]1\dfrac{1}{5}\times 2\dfrac{2}{3}[/latex]
Step 1: [latex]1\dfrac{1}{5}=\dfrac{6}{5}[/latex] and [latex]2\dfrac{2}{3} = \dfrac{8}{3}[/latex]
Step 2: No whole numbers by themselves.
Step 3: Question is rewritten as [latex]\dfrac{6}{5}\times\dfrac{8}{3}[/latex]
Step 4 & 5: [latex]\dfrac{\cancel{6}2}{5}\times\dfrac{8}{\cancel{3}1}=\dfrac{16}{5}[/latex] (an improper fraction)
Step 6: [latex]\dfrac{16}{5} = 3 \dfrac{1}{5}[/latex] [latex]\begin{array}{r}3\\ 5\enclose{longdiv}{16}\\15\\ \hline 01\end{array}[/latex]
[latex]1\dfrac{1}{5}\times\dfrac2{2}{3}=3\dfrac{1}{5}[/latex]
Example T
[latex]4\times 2\dfrac{5}{6}[/latex]
Step 1 and 2: [latex]4 = \dfrac{4}{1}[/latex] and [latex]2 \dfrac{5}{6}= \dfrac{17}{6}[/latex]
Step 3: The question is rewritten as [latex]\dfrac{4}{1}\times\dfrac{17}{6}[/latex]
Step 4, 5 and 6: [latex]4\times 2\dfrac{5}{6}[/latex] = [latex]\dfrac{\cancel{4}2}{1}\times\dfrac{17}{\cancel{6}3}[/latex] = [latex]\dfrac{34}{3}[/latex] = [latex]11\dfrac{1}{3}[/latex]
Remember to only skip steps when you are totally confident in your method.
Writing out the steps will help you to get the answer right more often.
Exercise 6
Find the products
- [latex]2 \dfrac{1}{2}\times 6 =\dfrac{7}{\cancel{2}1} \times \dfrac{\cancel{6}3}{1} = 21[/latex]
- [latex]3 \times \dfrac{1}{5}=\dfrac{3}{1} \times \dfrac{6}{5} = \dfrac{18}{5} = 3 \dfrac{3}{5}[/latex]
- [latex]\dfrac{3}{14}\times 2\dfrac{1}{6}\times 12 =[/latex]
- [latex]3\times 4\dfrac{1}{3}=[/latex]
- [latex]1\dfrac{2}{5} \times 15 =[/latex]
- [latex]3\dfrac{3}{8} \times 8 =[/latex]
- [latex]4\times 2\dfrac{1}{2} =[/latex]
- [latex]2\dfrac{1}{4}\times 8 =[/latex]
Answers to Exercise 6
- [latex]5 \dfrac{4}{7}[/latex]
- 13
- 21
- 27
- 10
- 18
Exercise 7
This is extra practice if you feel you need it.
- [latex]1 \dfrac{ 1}{2}\times\dfrac{2}{3} =[/latex]
- [latex]1 \dfrac{1}{4}\times 3 \dfrac{1}{2} =[/latex]
- [latex]\dfrac{1}{3}\times 5 \dfrac{1}{2} =[/latex]
- [latex]1 \dfrac{1}{6}\times1 \dfrac{5}{7}=[/latex]
- [latex]\dfrac{1}{2}\times 2 \dfrac{1}{2}=[/latex]
- [latex]7 \dfrac{1}{4}\times 3 \dfrac{1}{3} =[/latex]
- [latex]7 \dfrac{1}{3}\times\dfrac{3}{8} =[/latex]
- [latex]2 \dfrac{1}{4}\times 3 \dfrac{1}{2} =[/latex]
Answers to Exercise 7
- 1
- [latex]4 \dfrac{3}{8}[/latex]
- [latex]1 \dfrac{5}{6}[/latex]
- 2
- [latex]1 \dfrac{1}{4}[/latex]
- [latex]24 \dfrac{1}{6}[/latex]
- [latex]2 \dfrac{3}{4}[/latex]
- [latex]7 \dfrac{7}{8}[/latex]
Problems using Multiplication of Common Fractions
The following exercise gives some typical word problems for multiplication of fractions. The type of wording is similar for decimals and common fractions.
Remember this important word:
A fraction of some number means to multiply
Example:
[latex]\dfrac{2}{3}[/latex] of her money means [latex]\dfrac{2}{3}[/latex] × amount of the money
[latex]\dfrac{1}{4}[/latex] of the children means [latex]\dfrac{1}{4}[/latex] × number of children
These techniques may help you through the problem-solving steps when you’re working with common fractions:
- Look for key words
- Look for familiar patterns in the wording.
- Round fractions to whole numbers to estimate and to try to make sense of the problem.
- Draw a sketch or diagram of what the problem is describing
List key words which point to multiplication:
Exercise 8
- Maria was angry with her children because they had eaten 2/3 of the 24 cupcakes she had made to take to a meeting. How many cupcakes did the kids eat?
- Double this recipe for Awesome Chocolate Chip Cookies.
- [latex]\dfrac{2}{3}[/latex] cup butter
- [latex]\dfrac{2}{3}[/latex] peanut butter
- [latex]\dfrac{5}{8}[/latex] white sugar
- [latex]\dfrac{5}{8}[/latex] brown sugar
- 2 eggs
- 1 [latex]\dfrac{3}{4}[/latex] tsp vanilla
- 1 [latex]\dfrac{1}{2}[/latex] cup flour
- 1 cup rolled oats
- 1 tsp baking soda
- [latex]\dfrac{1}{4}[/latex] tsp salt
- [latex]\dfrac{7}{8}[/latex] chocolate chips
Cream butter and peanut butter well, blend in sugar. Beat in eggs and vanilla. Add dry ingredients, blending very thoroughly. Add chocolate chips and mix. Drop by spoonful onto cookie sheet; cookies will flatten during cooking. Bake in 350 F° oven for 10 to 12 minutes.
- Marni is trying to gradually cut down the amount of coffee she drinks. Right now, she allows herself [latex]\tfrac{3}{4}[/latex] of a cup of coffee at breakfast, [latex]\tfrac{3}{4}[/latex] cup at morning break time, [latex]\tfrac{3}{4}[/latex] cup at lunch, and another [latex]\tfrac{3}{4}[/latex] cup after dinner. How many cups of coffee is she drinking per day right now?
- Sam’s truck uses [latex]\tfrac{1}{3}[/latex] of a tank of gas every time he drives to his girlfriend’s house. His tank holds 75 litres. How many litres of gas does he use to drive to his girlfriend’s place?
- If you do math for [latex]1\tfrac{1}{2}[/latex] hours every day you are at school, how many hours do you spend on math per month if you come to school twenty days in a month?
- David was complaining that his car insurance was the same price as [latex]\tfrac{7}{8}[/latex] of the cost of his car! His car cost $1200. What did he pay for car insurance?
- Justine was building a dog shed. She spent [latex]\tfrac{1}{3}[/latex] of an hour on the project every evening for 8 days in a row. How much time did she spend on the project?
Answers to Exercise 8
- 16 cupcakes
- Recipe for Awesome Chocolate Chip Cookies (Doubled)
- [latex]1 \dfrac{1}{3}[/latex] cup butter
- [latex]1 \dfrac{1}{3}[/latex] cup peanut butter
- [latex]1 \dfrac{1}{4}[/latex] cup white sugar
- [latex]1 \dfrac{1}{4}[/latex] cup brown sugar
- 4 eggs
- [latex]3 \dfrac{1}{2}[/latex] teaspoons vanilla
- 3 cups flour
- 2 cups rolled oats
- 2 teaspoons baking soda
- [latex]\tfrac{1}{2}[/latex] teaspoon salt
- [latex]1 \dfrac{3}{4}[/latex] cup chocolate chips
- 3 cups
- 25 L
- 30 hours
- $1050.00
- [latex]2\tfrac{2}{3}[/latex] hours
Topic A: Self-Test
Mark /20 Aim 16 / 20
- Find the products. 10 marks
- [latex]\dfrac{1}{4}\times\dfrac{3}{8}=[/latex]
- [latex]\dfrac{3}{8}\times\dfrac{5}{6} =[/latex]
- [latex]\dfrac{7}{9}\times\dfrac{3}{14}=[/latex]
- [latex]\dfrac{1}{4}\times 12 =[/latex]
- [latex]5\times\dfrac{4}{9}=[/latex]
- [latex]1\dfrac{1}{4}\times\dfrac{4}{5}=[/latex]
- [latex]\dfrac{3}{5} \times 7\dfrac{2}{9}=[/latex]
- [latex]6\times 1\dfrac{2}{3}=[/latex]
- [latex]1\dfrac{1}{2}\times 1\dfrac{3}{5}=[/latex]
- [latex]2\dfrac{7}{8}\times1\dfrac{9}{10}=[/latex]
- Solve the following word problems. 10 Marks
- Frank is hosting a big party. He needs to multiply his favourite tomato sauce by five to make enough to serve his guests. Five times this recipe for him. 4 Marks
- [latex]1\dfrac{1}{2}[/latex] Tbsp olive oil
- 1 onions
- [latex]\dfrac{1}{2}[/latex] tsp salt
- 2 bay leaves
- 6 garlic cloves
- [latex]\dfrac{1}{3}[/latex] tsp red chili flakes
- [latex]1\dfrac{3}{4}[/latex] diced tomatoes
- [latex]1\dfrac{1}{4}[/latex] brown sugar
- Joey practices playing the trumpet ¾ of an hour each day. How much time does he spend practicing each week? 2 Marks
- A Haida longhouse measures 15 [latex]\tfrac{1}{4}[/latex] m by 18 [latex]\tfrac{1}{3}[/latex] m. What area of land does it cover? 2 Marks
- Find the area of the rectangle. 2 Marks
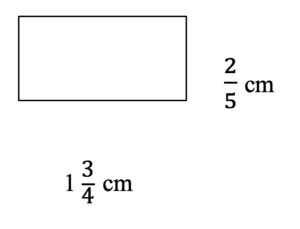
- Frank is hosting a big party. He needs to multiply his favourite tomato sauce by five to make enough to serve his guests. Five times this recipe for him. 4 Marks
Answers to Topic A Self Test
-
- [latex]\dfrac{3}{32}[/latex]
- [latex]\dfrac{5}{16}[/latex]
- [latex]\dfrac{1}{6}[/latex]
- 3
- [latex]2\dfrac{2}{9}[/latex]
- 1
- [latex]4\dfrac{1}{3}[/latex]
- 10
- [latex]2\dfrac{2}{5}[/latex]
- [latex]5\dfrac{37}{80}[/latex]
-
-
- [latex]7\dfrac{1}{2}[/latex] Tbsp olive oil
- 5 onions
- [latex]2\dfrac{1}{2}[/latex] tsp salt
- 10 bay leaves
- 30 garlic cloves
- [latex]1\dfrac{2}{3}[/latex] tsp red chili flakes
- [latex]8\dfrac{3}{4}[/latex] diced tomatoes
- [latex]6\dfrac{1}{4}[/latex] brown sugar
- [latex]5\dfrac{1}{4}[/latex] hours
- [latex]279\dfrac{7}{12}[/latex] m2
- [latex]\dfrac{7}{10}[/latex] cm2
-
In mathematics, a symbol that tells what operation is to be performed or what the relationship is between the numbers.
+ plus, means to add
− minus, means to subtract
× multiplied by, "times"
÷ divided by, division
= equal, the same quantity as
≠ not equal
≈ approximately equal
< less than
> greater than
≤ less than or equal to
≥ greater than or equal to

