2 Average Speed & Velocity
Resources
- Watch: Mechanical Universe – Episode 5 – Vectors
- Extra Help: Distance and Displacement
- Extra Help: Speed and Velocity
2.1 Average Speed and Velocity
Speed and velocity are both measures of how quickly something (car, person, jet, etc.) moves from one place to another[1]. While people often use these interchangeably, they are different measures of the same phenomenon and require looking at the same problem in different ways.
Suppose someone asks several of his friends to help move a heavy refrigerator roughly 5 m, for a reward of pizza and beer afterwards. This may sound straightforward, but one important detail is left out: this refrigerator must be moved to an apartment directly above the one it is in. The friends soon understand that the total distance the refrigerator is to be moved is a lot greater than the described roughly 5 m. In this instance, the refrigerator is to be moved about 40 m down the hallway, up the stairs to the second floor (a vertical distance of 5 m) and then 40 m down the hall into the other apartment.
The friend was honest in their original description of the move: technically, the refrigerator needed to be moved 5 m straight up. However, this 5 m straight upwards would be measured as a displacement. The total distance travelled in this example would be 40 m + 40 m + 5 m + the distance up the flight of stairs.
Measures of distance moved are thus defined as the total distance travelled, where the change in displacement is the change in position that includes the direction between these two positions.
These measures are also classified as scalar and vector measures, with the scalar just being a number of how much and the vector (it generally has an arrow over top the symbol) giving a change in the measure and including the direction involved.
Because of this, you will encounter two sets of measures in introductory physics, a scalar and a vector that can be used to measure the same phenomenon. We start this off by looking at the average speed and the average velocity of an event.[2]
[latex]v=\dfrac{\Delta d}{t}[/latex] [latex]\vec{v}=\dfrac{\Delta\vec{d}}{t}[/latex]
Where…
- [latex]v[/latex] is the speed, commonly measured in metres/second (m/s) or kilometres/hour (km/h)
- [latex]\vec{v}[/latex] is the velocity, commonly measured in metres/second (m/s) or kilometres/hour (km/h) and includes a direction
- [latex]\Delta d[/latex] is the distance travelled, commonly measured in metres (m), kilometres (km)
- [latex]\Delta\vec{d}[/latex] is the change in displacement, commonly measured in metres (m), kilometres (km) and includes a direction
- [latex]t[/latex] is the time, commonly measured in second (s) or hours (h)
Often speed and velocity involve combining several different motions to calculate the average speed or velocity of a grouping of linked motions.
Scalar is the quantity of a measure and is only a magnitude without other data.
Vector is a measure that has an associated direction. It typically refers to magnitude and directional change from the initial to the final measurement.
Two of these measures: time and distance/displacement, are classified as base units in the International System of Units.[3]
Time
Prior to 1900, time was defined as the fraction, [latex]\tfrac{1}{86\;400}[/latex] of an Earth day (24 h × 60 min × 60 s). This was redefined shortly thereafter (1956-1967) as the fraction [latex]\tfrac{1}{31\;556\;925.9747}[/latex] of the tropical year of 1900, January 0 at 12 hours ephemeris time. Time is currently (since 1967) defined by the energy emitted/absorbed by the caesium-133 atom.
Depending on what fields you study in the future, you will encounter numerous measures of time, the largest of which is the galactic year, which is the time based on one complete rotation of our galaxy. One galactic year is currently given as 230 million years.
Historically the measurement of time presented early scientists with a number of challenges. Originally the Egyptian calendar of one year was defined by measurements based on the heliacal rising of the binary star Sirius, marking the onset of the flooding of the Nile River.[4] Many subsequent civilizations, including the Babylonians, Sumerians, Greeks and the Maori, based their calendars on the phenomenon of heliacal rising, correcting previous calendars’ lack of a leap year. These corrected calendars were replaced with the current Julian (proposed by Julius Caesar in 46 BCE) and Alexandrian (based on the early Egyptian calendar) calendars. Currently the Gregorian calendar, a restructuring of the Julian calendar, is the one most commonly used.
The hourglass and the water clock were early devices that measured time.[5] The hourglass (sandglass, sand timer, sand clock or egg timer) is recorded in history as far back as 1600s BCE in Babylonia and Egypt. These early hourglasses consisted of two connected glass bulbs, one filled with sand that flowed freely to the other when the sand-filled bulb was positioned above the other. While common throughout the ancient Middle East, records of its arrival in Europe indicate its presence from the mid-1300s onward. The hourglass continued in common usage until the 1500s, when it began to decline in popularity as a timing device due to the technological development of mechanical clocks.
Water clocks are claimed to have been present in China as early as 4000 BCE, and it is known that water clocks were present in Egypt around the 1600s BCE. The design of a water clock is simple: water flows slowly from an upper source to a container where the amount of water and therefore time can be measured. Both hourglasses and water clocks depend on the rate of flow of either sand or water to indicate the passage of time.
The image to the right is of the oldest surviving water clock from Egypt, 1400 BCE. The image below is of water clock calculations by Nabû-apla-iddina (600-500 BCE).
Galileo Galilei created a time measuring device called the pulsilogon, which he based on the regular timing of a swinging pendulum. One of his first applications of the pulsilogon was to measure a person’s pulse, by lengthening or shorting the pendulum until the time for one swing of the pendulum (called the period) matched the pulse rate. Medical historians view this invention as the birth of precision in medicine. Using this pendulum time piece, Galileo discovered some of his foundational concepts in physics.
Length
The measurement of length originates in multiple cultures and civilizations. Common English measures such as the inch (length of a thumb), the foot (the length of a foot), the yard (length of an average pace) and the mile (one thousand of the Roman concept of two steps to a pace) all have historical origins. Most common of all these measures for length was that of the cubit, which was measured as the distance between the elbow and the tip of the middle finger. As you can remember from chapter one, there are multiples of measures used for length, each having specific uses for various trades and specialties.
The metric system of measures was created during the French Revolution in 1799, to clarify the definition of length and other measures. The early metric system, based on a decimal fraction of the distance from the Equator to the North Pole, was also used to define the kilogram as the mass of water contained in a cube of 10 cm length (decimetre). As time passed, the need for greater accuracy arose, resulting in the creation of two standard artifacts, created out of platinum and iridium, for the metre and the kilogram. These constructions were used to define length and mass, having since become common measures in all countries except the United States, Myanmar (Burma) and Liberia. The image to the right is the only surviving artifact of the original metre, and is located at 36, rue Vaugirard, Paris, France.
Prior to 1793, length was defined by the fraction 1/10000000 of the meridian length (through Paris) between the North Pole and the Equator. This also was redefined for a short time (1960-1983) by the wavelength of radiation emitted by the Krypton-86 atom. Length is currently defined (1983) by a Speed Equation: the distance that light travels in a vacuum in the fraction 1/299792458 seconds.
Using the speed equation: [latex]v = \dfrac{d}{t}[/latex], we can see that [latex]d = v t[/latex], which means for the base measure of length:
One meter (m) = (speed of light) (1/299792458 seconds).
The following example will help to explain the difference in measuring speed and velocity.
Example 2.1.1
Using the following sketch of a hike around a lake, solve for the following questions.
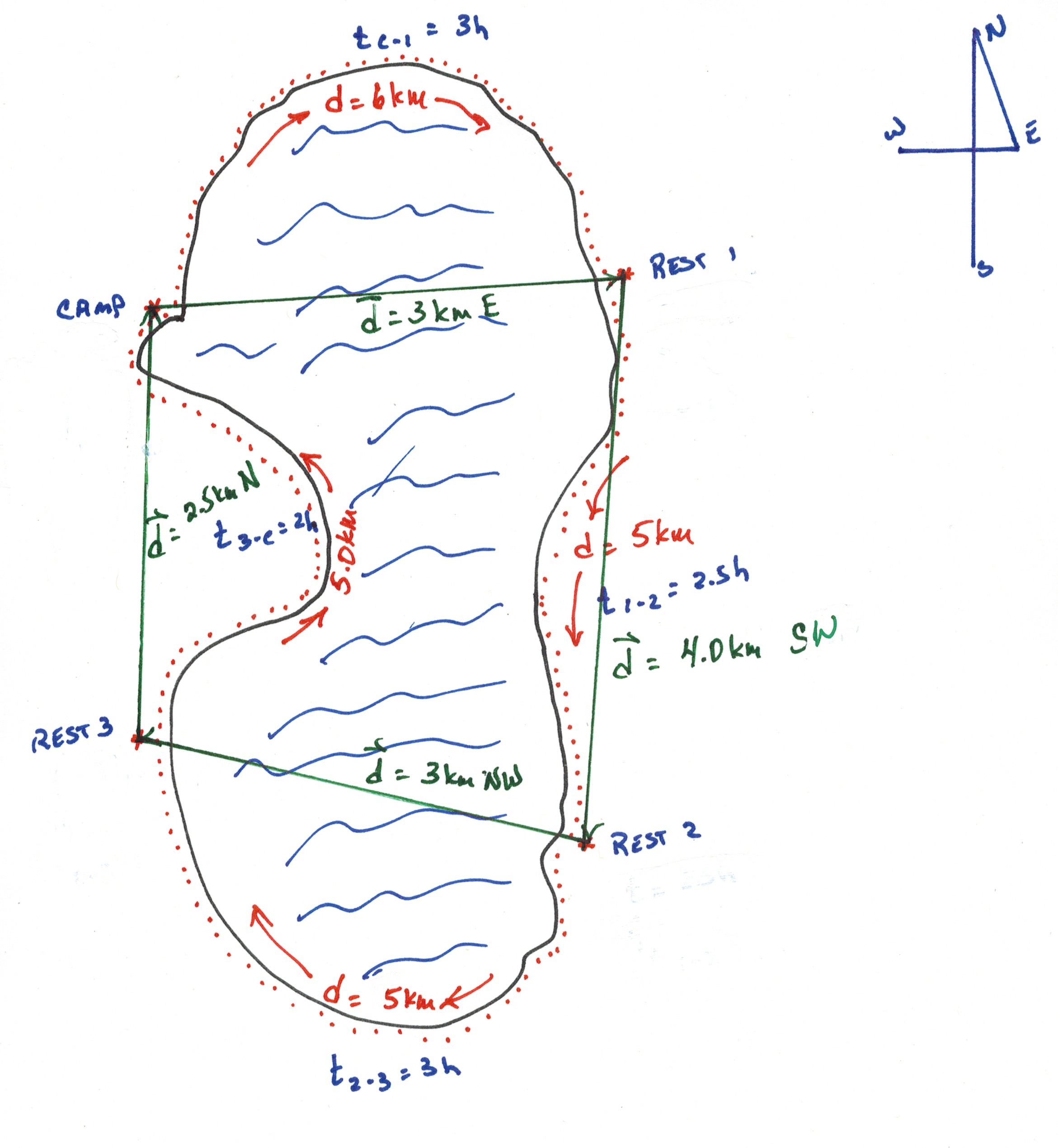
- What is the average speed and velocity in walking from the camp to the 1st rest spot?
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- [latex]\Delta d=6\text{ km}[/latex]
- [latex]t=3\text{ h}[/latex]
- [latex]v=\dfrac{6\text{ km}}{3\text{ h}}[/latex]
- [latex]v=2\text{ km/h}[/latex]
- [latex]\vec{v}=\text{find}[/latex] ([latex]\vec{v}=\dfrac{\vec{d}}{\text{t}}[/latex])
- [latex]\Delta\vec{d}=3\text{ km E}[/latex]
- [latex]t=3\text{ h}[/latex]
- [latex]\vec{v}=\dfrac{3\text{ km E}}{3\text{ h}}[/latex]
- [latex]\vec{v}=1\text{ km/h E}[/latex]
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- What is the average speed and velocity in walking from the 1st to the 2nd rest spot?[6][7]
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- [latex]\Delta d=5\text{ km}[/latex]
- [latex]t=2.5\text{ h}[/latex]
- [latex]v=\dfrac{5\text{ km}}{2.5\text{ h}}[/latex]
- [latex]v=2\text{ km/h}[/latex]
- [latex]\vec{v}=\text{find}[/latex] ([latex]\vec{v}=\dfrac{\vec{d}}{t}[/latex])
- [latex]\Delta\vec{d}=4.0\text{ km SW}[/latex]
- [latex]t=2.5\text{ h}[/latex]
- [latex]\vec{v}=\dfrac{4.0\text{ km SW}}{2.5\text{ h}}[/latex]
- [latex]\vec{v}=1.6\text{ km/h SW}[/latex]
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- What is the average speed and velocity in walking from the 2nd to the 3rd rest spot?
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- [latex]\Delta d=5\text{ km}[/latex]
- [latex]t=3\text{ h}[/latex]
- [latex]v=\dfrac{5\text{ km}}{3\text{ h}}[/latex]
- [latex]v=1.67\text{ km/h}[/latex]
- [latex]\vec{v}=\text{find}[/latex] ([latex]\vec{v}=\dfrac{\vec{d}}{t}[/latex])
- [latex]\Delta\vec{d}=3.0\text{ km NW}[/latex]
- [latex]t=3\text{ h}[/latex]
- [latex]\vec{v}=\dfrac{3\text{ km NW}}{3\text{ h}}[/latex]
- [latex]\vec{v}=1\text{ km/h NW}[/latex]
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- What is the average speed and velocity in walking from the 3rd rest spot back to the camp?
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- [latex]\Delta d=5\text{ km}[/latex]
- [latex]t=2\text{ h}[/latex]
- [latex]v=\dfrac{5\text{ km}}{2\text{ h}}[/latex]
- [latex]v=2.5\text{ km/h}[/latex]
- [latex]\vec{v}=\text{find}[/latex] ([latex]\vec{v}=\dfrac{\vec{d}}{t}[/latex])
- [latex]\Delta\vec{d}=2.5\text{ km N}[/latex]
- [latex]t=2\text{ h}[/latex]
- [latex]\vec{v}=\dfrac{2.5\text{ km N}}{2\text{ h}}[/latex]
- [latex]\vec{v}=1.25\text{ km/h N}[/latex]
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- What is the average speed and velocity walking the entire hike? (Do not include the time taken to rest.)
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- [latex]\Delta d=21\text{ km}[/latex]
- [latex]t=10.5\text{ h}[/latex]
- [latex]v=\dfrac{21\text{ km}}{10.5\text{ h}}[/latex]
- [latex]v=2\text{ km/h}[/latex]
- [latex]\vec{v}=\text{find}[/latex] ([latex]\vec{v}=\dfrac{\vec{d}}{t}[/latex])
- [latex]\Delta\vec{d}=0\text{ km}[/latex]
- [latex]t=10.5\text{ h}[/latex]
- [latex]\vec{v}=\dfrac{0\text{ km}}{10.5\text{ h}}[/latex]
- [latex]\vec{v}=0\text{ km/h}[/latex]
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
Final change in displacement = 0 km. The hikers ended up at the same position that they started from.
Example 2.1.2
What is the average speed and velocity of a hiker who walks 15 km (displacement change of 3.0 km NE) in 5.0 h?
- [latex]v=\text{find}[/latex] ([latex]v=\dfrac{d}{t}[/latex])
- [latex]\Delta d=15\text{ km}[/latex]
- [latex]t=5.0\text{ h}[/latex]
- [latex]v=\dfrac{15\text{ km}}{5\text{ h}}[/latex]
- [latex]v=3.0\text{ km/h}[/latex]
- [latex]\vec{v}=\text{find}[/latex] ([latex]\vec{v}=\dfrac{\vec{d}}{t}[/latex])
- [latex]\Delta\vec{d}=3.0\text{ km NE}[/latex]
- [latex]t=5.0\text{ h}[/latex]
- [latex]\vec{v}=\dfrac{3.0\text{ km NE}}{5\text{ h}}[/latex]
- [latex]\vec{v}=0.60\text{ km/h NE}[/latex]
Example 2.1.3
What is the possible area covered by a person who walks 100 m in an unknown direction?
Solution
This question is different than the two previous examples as it does not require a speed or velocity calculation. Rather it is a geometry question, specifically the area of a circle of radius 100 m.

- [latex]\text{A}=\pi r^2[/latex]
- [latex]\text{A}=\pi(100\text{ m})^2[/latex]
- [latex]\text{A}=10000\pi\text{ m}^2[/latex]
- [latex]\text{A}=31400\text{ m}^2[/latex] (three digits accuracy)
Exercise 2.1.1 Average Speed & Velocity
- What must be your average speed, to travel 684 km in 9.0 h?
- When Donovan Bailey ran the 100 m in 9.84 s, what was his average speed?
- A cruise ship moves at an average speed of 25 km/h.
- What distance does it travel in a day?
- What amount of time does it need to travel 800 km?
- A person walks around a store traveling 65 m in 155 s but only changes their displacement by 18 m NW. What is the person’s average speed and velocity?
- You are given a task to find a puppy who wandered 600 m in an afternoon from your back yard. What size search area would you need to check?
2.2 Average Speed/Velocity (Converting Units)
In real life, you will often need to convert from multiple units to a shared common unit to be able to solve a problem. For speed, you may be given speeds in m/s, cm/s, km/h, km/s, length in m, cm, km, light years and time in seconds, minutes, hours, days or years. Make sure that all units are the same measure before you start solving the problem; this will make the task significantly easier.
Example 2.2.1
What must be your average speed in km/h to travel 10.4 km in 90 minutes?
You can see that we have two different measures in this question, distance travelled in km and time measured in minutes with the final answer to be in km/h. Therefore, the time must be converted to hours.
Data:
- [latex]v=\text{find}[/latex]
- [latex]\Delta d-10.4\text{ km}[/latex]
- [latex]t=90\text{ minutes or }1.5\text{ hours}[/latex]
First: 90 minutes is divided by 60 minutes to convert to hours. Therefore 90 minutes = 1.5 hours.
- Now: [latex]v=\dfrac{d}{h}[/latex]
- [latex]v=\dfrac{10.4\text{ km}}{1.5\text{ h}}[/latex]
- [latex]v\approx6.9\text{ km/h}[/latex]
Example 2.2.2
If a distracted driver traveling at 60 km/h looks at their cell phone for 9.0 s, how far have they driven without looking at the road?
You have a choice to convert the time to hours or the speed to m/s. It is easier to convert the speed (remember this conversion factor: 1 m/s = 3.6 km/h).
Data:
- [latex]\Delta\text{d}=\text{find}[/latex]
- [latex]v=60\text{ km/h or }16.7\text{ m/s}[/latex]
- [latex]t=9.0\text{ s}[/latex]
Now: [latex]v=\dfrac{d}{t}[/latex] or [latex]d=v t[/latex]
[latex]d=(16.7\text{ m/s})(9.0\text{ s})[/latex]
[latex]d=150\text{ m}[/latex]
Example 2.2.3
What distance is covered by a person who drives an average speed of 50 km/h in the city for 40 minutes and then drives at an average speed of 110 km/h for 1 hour and 20 minutes?
Data:
- [latex]\Delta d=\text{find}[/latex]
- [latex]v=50\text{ km/h}[/latex]
- [latex]t=40\text{ minutes}[/latex]
First convert 40 minutes to hours (divide by 60 minutes = 1 hour).
[latex]40\text{ minutes}\div60=\dfrac{2}{3}\text{ h}[/latex]
Now: [latex]v=\dfrac{d}{t}[/latex] or [latex]d=v t[/latex]
[latex]d=(50\text{ km/h})(\frac{2}{3}\text{ h})=33.3\text{ km}[/latex]
- [latex]\Delta d=\text{find}[/latex]
- [latex]v=110\text{ km/h}[/latex]
- [latex]t=1\text{ hour }20\text{ minutes}[/latex]
Need to convert 1 hour 20 minutes to hours.
[latex]1\text{ hour }20\text{ minutes}=1\dfrac{1}{3}\text{ or }\dfrac{4}{3}\text{ hours}[/latex]
Now: [latex]v=\dfrac{d}{t}[/latex] or [latex]d=v t[/latex]
- [latex]d=(110\text{ km/h})(\frac{4}{3}\text{ h})=146.7\text{ km}[/latex]
Therefore the total distance travelled is:
[latex]d_{total}[/latex] = 33.3 km + 146.7 km
[latex]d_{total}[/latex] = 180 km
Exercise 2.2.1
- Show the conversion factor between metres/second and kilometres/hour.
- If you are driving 90 km/h and look to the side for 3.0 s, how far would you travel in this inattentive period?
- What distance will an auto travel in 55 minutes while traveling at 110 km/h?
- A killer tomato[8] can travel at 24 km/h. What distance could it travel in 1 hour & 15 minutes?
- A model aircraft can fly at 30 m/s. If it breaks free from radio control, what distance could it fly, if it has enough fuel for 2 hours & 20 minutes of flight?
- Two transport aircraft take off at the same time headed on the same flight path. One travels at 240 km/h for 3 hours 40 minutes before it lands and the other travels at 90 m/s for 2 hours 55 minutes before landing. How far apart are the planes after they have landed?
Exercise 2.2.2
These take more time to solve. Best to grab a coffee or so to do these following questions. They are meant to make you think.
- In a simulation of a search and rescue, a person is assumed to walk at 4 km/h, in an unknown direction. How big is the search area one would need to cover after this person has been walking for 8 h? (You need to use the area of a circle for this problem.)
- A Boeing 747-400 whose cruising speed is 918 km/h (mach 0.855) travels a distance of 1995 km in 2.5 h. What was the average speed of the headwind slowing this jet?
- An airplane travels 1600 km at a speed of 1000 km/h. It then runs into a headwind that slows it to 875 km/h for the next 2100 km. What was the average speed of the plane for this trip?
- A passenger train travels at 80 km/h for 1/2 hour, and then after leaving city limits, 100 km/h for 2 hours.
- How far did it travel?
- What was its average speed for this trip?
- A rally car driver drives the first 100 kilometres of a 200 kilometre race at an average speed of 30 km/h. What must be the average speed of this driver on the return trip to have an overall average speed for the entire race of 60 km/h?
- What amount of time does it take an echo to return to a person standing 100 m away from a cliff? (Use a speed of sound of 350 m/s)
- A brother and sister both start from home at the same time and drive to work 40 km distant. The posted speed for the road they travel is 110 km/h. If the sister speeds by 10 km/h and the brother travels 10 km/h under the speed limit, by what difference in time will they arrive at work?
- A person’s left and right palms are moving toward each other at a speed of 4.0 m/s each. A fast flying insect originally on the left palm flies to the right palm at 10 m/s; when it arrives there it immediately turns back to the left palm flying at the same speed. It continues to fly back and forth between the palms (originally separated by 1.0 m) until the end. What is the total distance travelled by the insect?
2.3 Relative Velocity in Two Dimensions
In real life, one often encounters motion on or in a moving medium. Common examples of this include: one walking on an escalator, a boat moving on a stream or river, and an aircraft that is flying into moving air such as the jet stream.
The simplest way to understand this is to relate it to a common experience in which most of us have participated: walking in or opposite to the direction that an escalator is moving.
When walking in the same direction as the escalator is moving, we travel faster. To calculate our relative speed, we add the speed of the escalator to the speed of our walking pace. If we wish to put this in an equation, it looks like the following:
[latex]v_{\text{relative}} = v_{\text{escalator}} + v_{\text{walking}}[/latex]
When walking in the opposite direction as the escalator is moving, we travel slower. To calculate our relative speed, we subtract the speed of the escalator from the speed of our walking pace. If we wish to put this in an equation, it looks like the following:
[latex]v_{\text{relative}} = v_{\text{escalator}} - v_{\text{walking}}[/latex]
Should we not walk fast enough, we could easily end up moving backwards, even though we are walking forwards.[9]
This is slightly more complicated for aircraft and watercraft that are moving in air or water that itself is moving. If moving in the same direction or the opposite direction that the air or water is moving, we generally add or subtract these two velocities, as we did above for walking on an escalator. If moving at an angle to the direction the air or water is moving then one generally draws a sketch and uses trigonometry to find the relative velocity.
This is done by using either right angle trigonometry and the Pythagorean Theorem or non-right angle trigonometry. The equations to use for these are as follows:
- For any right triangle a, b and c:

[latex]a^2 + b^2 = c^2[/latex]
- For any non-right triangle a, b and c:
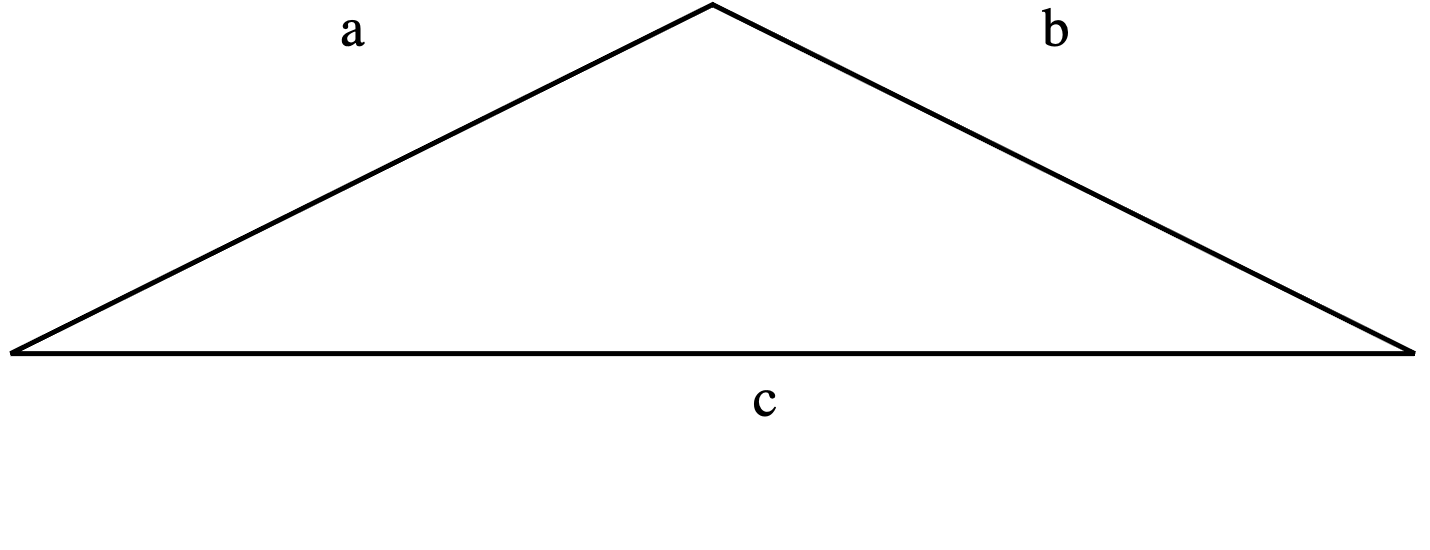
[latex]a^2 = b^2 + c^2 - 2bc \cos(A)[/latex]
[latex]b^2 = a^2 + c^2 - 2ac \cos(B)[/latex]
[latex]c^2 = a^2 + b^2 - 2ab \cos(C)[/latex]
Where…
- [latex]\text{Sin} = \dfrac{\text{Opposite}}{\text{Hypotenuse}}[/latex]
- [latex]\text{Cos} = \dfrac{\text{Adjacent}}{\text{Hypotenuse}}[/latex]
- [latex]\text{Tan} = \dfrac{\text{Opposite}}{\text{Adjacent}}[/latex]

The Crimes of Galileo
It is challenging today to find anyone who was not told at an early age that the Earth orbits around the Sun; this is the heliocentric theory. However, if we go back about four centuries, one of the greatest scientists of his time, Galileo Galilei (1564-1642), was threatened with torture and nearly executed for teaching such a doctrine.
Centuries earlier, two Greek philosophers, Eratosthenes (276-194 BCE) and Aristarchus (310-230 BCE), espoused the same theory. Using astronomy and geometry, they obtained reasonably accurate measures of the size of the Earth and the Moon, and calculated the distances from the Earth to the Moon and the Sun. Despite the existence of this theory, Ptolemy (100-170 CE) created an elaborate model of the universe, using what he described as crystalline spheres on which all known heavenly bodies moved. The Ptolemaic system of explaining the motions of the universe survived for the next fourteen centuries.
In 1530, Nicolaus Copernicus (1473-1543) challenged the Ptolemaic model with a heliocentric theory that placed the Sun in the centre of the solar system, orbited by the planets. At the time, people believed that if the Earth rotated, it would fly apart, flinging pieces of the planet far and wide in the opposite direction of the Earth’s rotation. Added to the prevailing Christian doctrine of man as God’s greatest creation, who therefore must be at the centre of the universe, this general lack of understanding of relative motion created opposition to Copernicus’s theory.
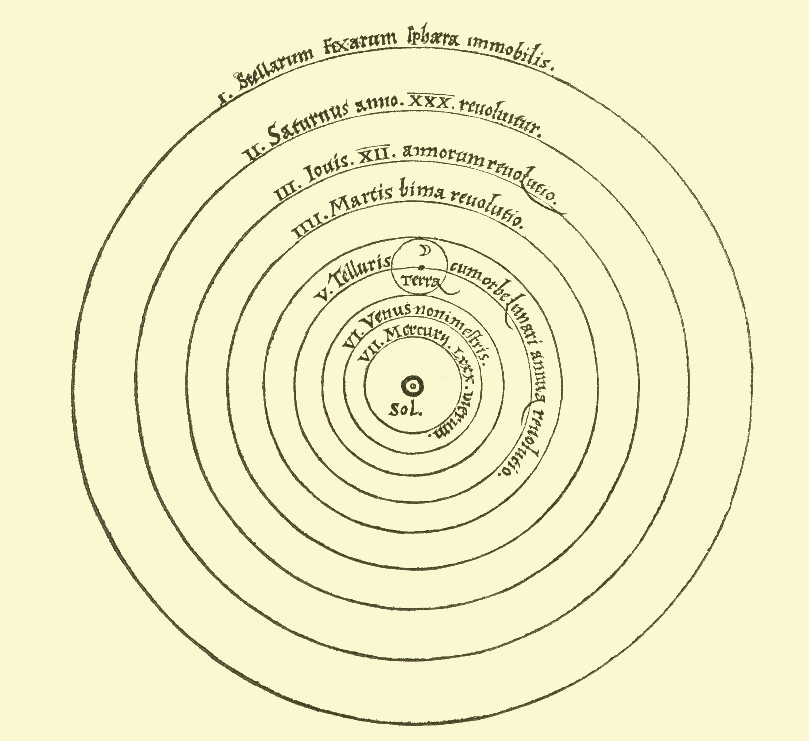
Copernicus asked why only the Earth would be comprised of the Aristotelian elements of earth, water, air and fire, when all objects in the heavens were composed of the quintessence.[10] One of Copernicus’s supporters, the Dominican friar Giordano Bruno (1548-1600), expanded upon the Copernican model, declaring that stars were spread throughout infinite space, and positing the possibility of other inhabited worlds. While Bruno, who was burned at the stake, paid with his life for his belief in the accuracy of the heliocentric model, it was Galileo Galilei’s technological advancement, the telescope, that suggested that the Moon was quite similar to the Earth and therefore the known planets were also like the Earth and not composed of the quintessence.
Galileo’s understanding and refinement of the telescope, turning it from 3x magnification to over 9x magnification, won him immediate praise and financial reward from contemporary military leaders. Using the telescope, the military defenders of Venice[11] could detect the sails of advancing ships two or more hours before the ships could detect the city. While Galileo was a gifted and curious scientist, his financial burdens[12] outstripped his teaching salary from the University of Padua. Galileo was granted tenure at the university, with an increased salary, for the advancement of the telescope.
Galileo’s first discoveries with his improved telescope included mountains on the Moon, and on January 7, 1610, the four moons of the planet Jupiter.[13] Understanding the risks of his discovery, Galileo named the moons of Jupiter after members of the Medici family, the Dukes of Tuscany, and gifted the Grand Duke with a further improved 20x magnification telescope to be able to better see the moons named after his family members. Galileo’s political maneuver protected him in Venice, however, he was warned about venturing outside the city due to tension between the Church and the city-state. Venice was attempting to impose taxes on the Church, whose geocentric interpretation of the scriptures held that Man living on Earth was the centre of the universe.
In 1616 the Catholic Church[14] condemned the Copernican system, placing Galileo in danger of heresy for his stance on the heliocentric universe. Galileo soon increased this risk by not only continuing to defend his position but writing of his beliefs in a work called, “Dialogue Concerning the Two Chief Systems of the World-Ptolemaic and Copernican.” Confident in the validity of his work, Galileo had the characters in his book, including one named Simplicio, who was clearly named after the current Pope, discuss the merits of his position.
The order to cease publishing Galileo’s book, five months after its publication in March 1632, accompanied the order for Galileo to stand trial. Having been threatened in 1616[15] with torture in the dungeons below the Vatican, a tour of the torture chambers during his trial helped to convince Galileo to tone down the writings in his Dialogues. He spent his remaining years in penance under house arrest at his villa until his death in 1642.[16]
Ironically, the Vatican ordered Galileo’s sentence to be published widely in scientific circles. Historians have argued that this strong stance taken by the Catholic Church effectively discouraged the development of science in Italy for many generations afterwards.
Galileo’s “Two New Sciences”
Following the publication of his Dialogue Concerning the Two Chief Systems of the World-Ptolemaic and Copernican, the Vatican banned any further publication of any of Galileo’s works, including any future writing. Galileo wrote his final book, Discourses and Mathematical Demonstrations Relating to Two New Sciences (1638), while he was under house arrest. The Vatican ban resulted in failed attempts to publish his last work in France, Poland and Germany, but Lodewijk Elzevir finally published it in Holland. Only fifty copies were exported to Rome, all of which quickly sold.
While Two New Sciences was written in a similar form to Galileo’s earlier work, Two Chief Systems, the discussions continued between the familiar Simplicio, Sagredo and Salviati. Simplicio no longer had the simplistic, stubborn Aristotelian beliefs of the time, and represented Galileo’s early beliefs as he started his studies. Sagredo represented Galileo’s changing views, and Salviati represented Galileo’s final views on his research. Such a change in his writing may have helped Galileo to escape any further punishment beyond house arrest.
This important work paved the way for those who followed Galileo to understand relative motion.
Relative Motion
Aristotelian theory argues against the rotation or spin of the Earth, stating that an object dropped at the equator would land far behind the position from which it was dropped, e.g.: if the Earth is rotating at 1000 km/h or 278 m/s at the equator and the dropped object takes two seconds to reach the ground, it would land 556 m behind; an object taking 4 s to reach the ground would fall behind over a kilometre.
Galileo used the example of falling objects on a moving ship to explain why a falling object could fall straight down if the Earth was indeed spinning. His thought experiment compared what was expected to happen according to Aristotelian logic, to what can actually be observed to happen. Without actually conducting the experiment, anyone working on ships knew that falling objects fall straight down.[17] Galileo also proposed that if observed from the shore, the observer would see a projectile type motion. In this example, Galileo explained that objects in motion on moving surfaces would be observed differently by an observer on the moving surface and one standing on a surface that is stationary.
The following examples explore the algebra of relative motion
Example 2.4.1
What is the average speed of an airliner that has an air speed of 750 km/h flying directly into a jet-stream of 115 km/h?

Solution
The solution of this question is to subtract the speeds. They are in opposite directions:
- [latex]v[/latex] = 750 km/h − 115 km/h
- [latex]v[/latex] = 635 km/h
Example 2.4.2
What is the average speed of an airliner that has an air speed of 750 km/h flying in the same direction as a jet-stream of 115 km/h?

Solution
The solution of this question is to add the speeds. They are in the same directions:
- [latex]v[/latex] = 750 km/h + 115 km/h
- [latex]v[/latex] = 865 km/h
Example 2.4.3
What is the average velocity of an airliner that has an air speed of 750 km/h East flying in a jet-stream of 115 km/h South? Can you calculate the angle it is traveling at?
Solution
The solution for this question requires you to use the pythagorean theorem:
- [latex]v^2[/latex] = (750 km/h)2 + (115 km/h)2
- [latex]v^2[/latex] = 562500 km2/h2 + 13225 km2/h2
- [latex]v^2[/latex] = 575725 km2 /h2
Taking the square root of both sides gives us the speed:
- [latex]v[/latex] = 758.8 km/h or 759 km/h (sig correct)
Using the Tangent Function, the angle is 8.7°
Exercise 2.4
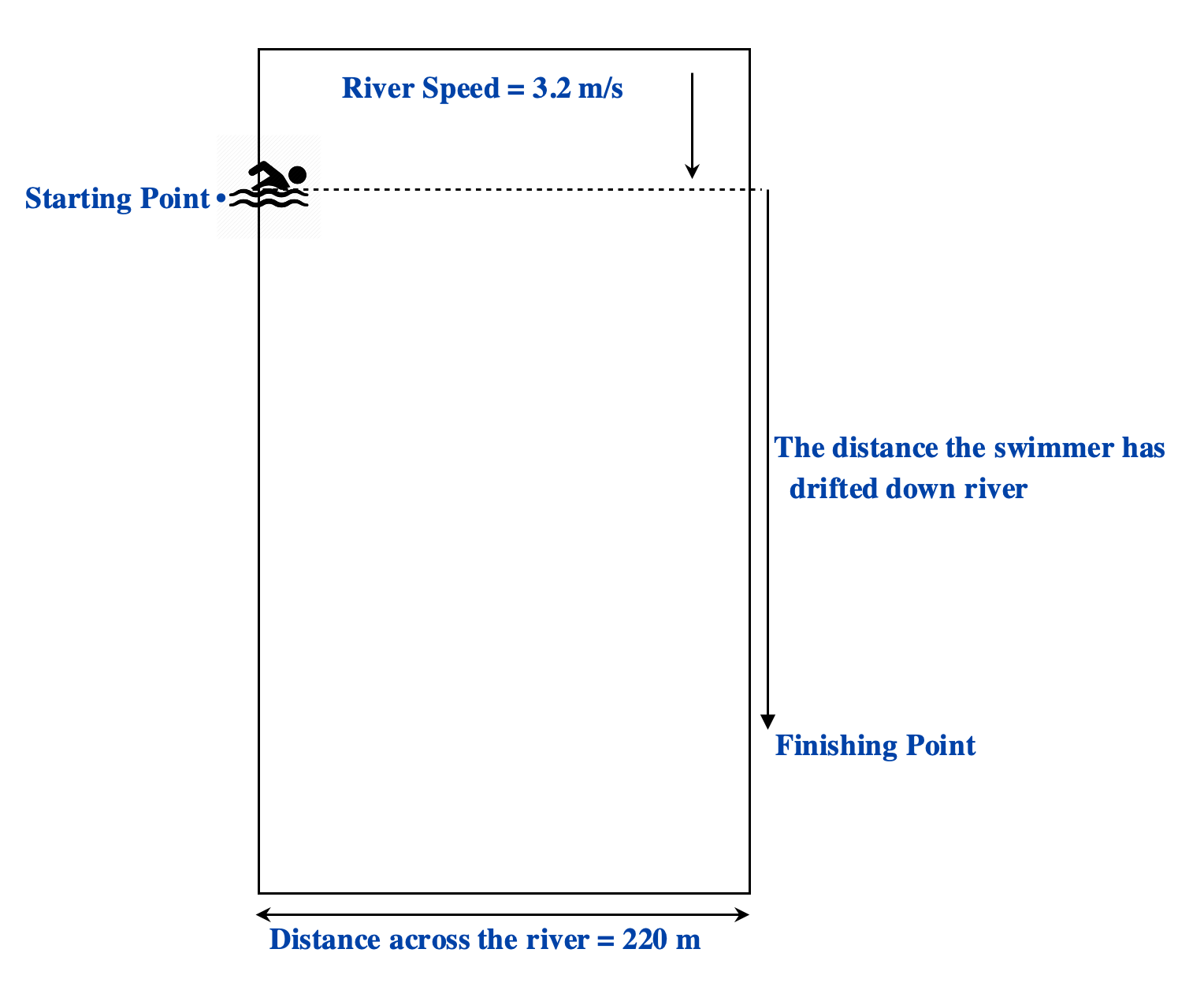
- One of the problems one encounters in real life is to swim or row across a river. In these cases the swimmer or rower swims at an angle across the river to maintain a straight path. This problem will look at the simplest form of this question, of swimming straight across the river and ending up drifting down from the starting point on the either side.In this problem, consider a swimmer that can swim at 1.0 m/s and who sets off to swim 220 m across a river which is flowing at 3.2 m/s. How far down river, will this swimmer have drifted by the time they have reached the other side?
- The Hindenburg class airships were 245 m long, 41 m wide (longer than 3 Boeing 747s parked end-to-end and only 24 m shorter than the Titanic). During their short commercial lifespan, the Hindenburg travelled 308323 km between Frankfurt, Rio De Janeiro and New York, carrying a total of 2798 passengers and 145 tonnes of freight and mail. Given that the maximum speed of the Hindenburg was 135 km/h, the speed of any wind it was flying into made for a significant impact on its travel.Consider the following:
- What is the average speed of the Hindenburg airship that has an air speed of 135 km/h flying directly into a jet-stream of 45 km/h?
- What is the average speed of a Hindenburg airship that has an air speed of 135 km/h flying in the same direction as a jet-stream of 45 km/h?
- What is the average velocity of a Hindenburg airship that has an air speed of 135 km/h East flying in a jet-stream of 45 km/h South? Can you calculate the angle it is traveling at?
- Suppose the Hindenburg needs to fly in a straight line for 250 km while encountering a 90° crosswind of 42 km/h. At what angle must it fly at to counteract the wind that is actively blowing it off course?
- What would its net ground speed towards its intended destination
- How much time would this take for this journey?
- The following shows a jet-stream forecast for Saturday 11th, 2014 that focuses on the Continental United States.
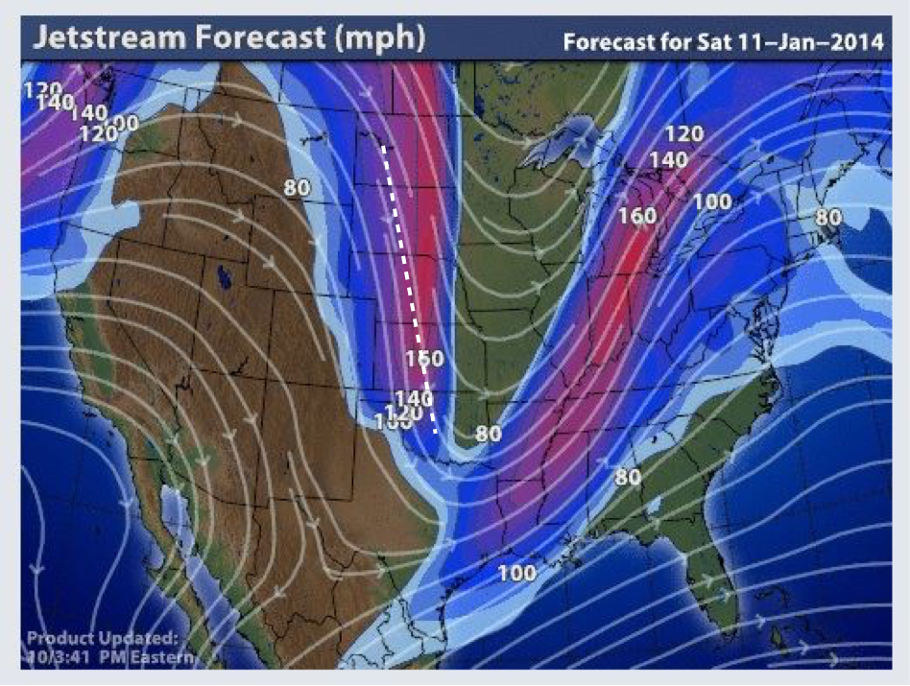
- What is length of the dashed line from X to X?
- Using a scale of 1 cm = 285 km, what distance does this represent?
- What is the expected speed of the jet-stream along this dashed line in km/h?
- If a Boeing 747-100B cruises at 893 km/h, how much time would it take to go from the Saskatchewan border to its destination just above Texas?
- For the return trip to Saskatchewan?
- Article to Read: Planes Are Ruining the Planet. New, Mighty Airships Won’t
- Article to Read: Jet Stream
- Article to Read: Flight from L.A. to London reaches 801 mph as a furious jet stream packs record-breaking speeds
Exercise 2.5.1: Horse Transportation
The people of the USA travelled around 8.8 × 1012 km in the year 2005. If only horses were used for transportation, then:
- How many horses would the USA need to provide transportation for its citizens? A horse can travel on average 32 km in 1 day.
- If the average horse needs 0.8 hectares of land to graze on for the year, then how many hectares would be needed to feed the horses required by the USA?
Exercise 2.5.2: The First Measure of the Speed of Light
The First Measure of the Speed of Light was a failure. Galileo recorded that he positioned himself on one hilltop with an assistant on a different hilltop to conduct the experiment. Galileo would open the shutter of his lantern; when the assistant saw the light from Galileo’s lantern they would open the shutter of their lantern. Galileo would then measure the time it took and, knowing the distance separating the two hilltops, he would be able to measure the speed of light. Galileo’s experiment had no chance of success. If we assume a half second reaction time for the assistant to open their lantern shutter, light would travel 150 000 km in that half second, a distance of 3.75 times around the Earth. If Galileo could have had an assistant on the Moon, light would have taken 2.56 s to travel back and forth between the Earth and the Moon, and even then we would need to add in the reaction time it takes to open the lantern shutter.
The breakthrough to measuring the speed of light came from observing the moon Io orbiting Jupiter.

While Galileo’s experiment failed, his suggestion that the orbital period of the four largest moons of Jupiter could be used as a kind of celestial clock was the key to measuring the speed of light. Discovered by Galileo in 1610, the Jovian satellite Io takes only 1.759 days to orbit the planet. It was Ole Römer (1644-1710) who, in making detailed recordings of the orbit of Io, noticed an error in his measurements. As the Earth moved in orbit around the Sun (and thus farther and closer to the planet Jupiter) the measured period of Io in orbit around Jupiter varied by some 22 minutes, i.e.: when the Earth was farthest away from Jupiter, Io’s orbit took 22 minutes longer than when the Earth was at its closest point to Jupiter. Römer’s interpretation is that it took 22 minutes for light to travel across the Earth’s orbit.
Questions:
- Given that the radius of the Earth’s orbit averages 1.49 x 1011 m calculate the speed of light using Römer’s value of 22 minutes (Christian Huygens (1629-1695) was the first to do this calculation).
- Later on, Römer’s measure of 22 minutes was found to be in error and was corrected to be 16.7 minutes. What would be the corrected measure of the speed of light using this data?
Exercise 2.5.3: The Time Delay in Communication between Earth and Mars
One of the challenges of exploring Mars from Earth, and in placing humans on the Martian surface, is the amount of time it takes for light or any other communication signal to travel between the two planets. The above sketch shows Mars and Earth in orbit around the Sun; while the Earth has nearly a circular orbit, Mars has more of an elliptical orbit. You will also notice that the units used in this sketch are given as A.U. (Astronomical Units) where 1 A.U. is defined as the average distance between the Earth and the Sun in its orbit[18].
1 A.U. = 149597870700 m
Since the distance measures between the Earth, Mars and the Sun in the above sketch are shown in A.U. we will need to convert all A.U. distances to metres (m).
One other factor that needs to be defined in working to solve the communication is that of the speed of light (c), which is the speed at which signals travel. All transmission communication, radio, television, radar, x-rays, gamma rays, infrared, ultraviolet radiation and light travel at the same speed, and are part of the same family called electromagnetic radiation (EM). The speed of light can be affected by the medium or object that the EM is traveling through but its maximum is in open space that is not affected by gravity. This speed shown by the symbol (c) is of great significance in the study of physics.
c = 299792458 m/s
Using the above data, find the minimum amount of time it takes it takes to send and receive a message sent from either planet for the following orbital separations between Mars and the Earth:
- [latex]d[/latex] = 0.38 A.U. (Martian Perihelion)
- [latex]d[/latex] = 0.66 A.U. (Martian Aphelion)
- [latex]d[/latex] = 2.66 A.U. (Maximum possible separation between the Earth and Mars)
Exercise 2.5.4: The Carrington Event
One of the challenges or dangers to Earth based technological devices is from interference from an intentional Electromagnetic Pulse or from the natural phenomenon of a Solar Flare from the Sun. Solar flares are powerful “Coronal Mass Ejections” (CMEs) composed of trillions of kilograms of charged electric particles traveling up to several million kilometres per hour through space. These solar flares are massive in size compared to the Earth and while they are not expected to cause any harm to life on Earth, they have the potential to destroy technological devices. Because of this threat, it is becoming increasingly relevant to have advance warning of such storms that are directed towards the Earth.
The largest recorded Solar Storm in history dates back to August 28, 1859 and has been named the Carrington Event (now identified as SOL 1859-09-01) after Richard C. Carrington (1826-1875), who both observed and recorded this CME. The effect of this coronal storm was so great that in some parts of the Earth people could read newspapers at night due to the brightness of the Northern Lights, and telegraph operators around the world reported being shocked or electrocuted by devices hooked up to the wires used for communication. In 2012, a CME similar in strength to the Carrington Event missed the Earth by nine days
Question: What was the speed of the CME ejected in the Carrington Event, if it took an estimated 17.6 hours to travel the distance between the Earth and the Sun? (d = 1.5 × 1011 m)
- Article to Read: NASA Developing Early Warning System for Solar Flares
- Article to Read: Byrd, D. (2012-12-09) Are Solar Storms dangerous to us?
- Research News: Lloyds (2013) Solar Storm risk to the North American Electric Grid
Exercise Answers
2.1 Average Speed/Velocity
- 76 km/h
- 10.2 m/s
-
- 600 km
- 32 h
-
- 0.42 m/s
- 0.12 m/s NW
- 1.13km2
2.2.1 Average Speed /Velocity (Converting Units)
- 1 m/s = 3.6 km/h. To change m/s to km/h multiply by 3.6 and to change km/h to m/s divide by 3.6
- 75 m
- 101 km
- 30 km
- 252 km
- 305 km
2.2.2 Average Speed and Velocity (Similar to 2.2.1 but these take more time to solve)
- 3220 km2 (≈ 3000 km2)
- 120 km/h
- 925 km/h
-
- 240 km
- 96 km/h
- Impossible to do
- 0.57 s
- 4.0 minutes
- 1.25 m
2.4 Relative Velocity in One Dimension
- 104 m
-
- 90 km/h
- 180 km/h
- 142 km/h, [latex]angle = 10^{\circ}[/latex]
- 18.1°
- 128 km/h
- 1.95 hours
-
- 6.3 cm
- 1800 km
- 256 km/h
- 1.57 h
- 2.81 h
2.5.1 Horse Transportation
- 7.53 × 108 Horses (753 million horses)
- 6.03 × 108 hectares
2.5.2 The First Measure of the Speed of Light
- 2.26 ×108 m/s
- 2.97 × 108 m/s
2.5.3 The Time Delay in Communication between Earth and Mars
- 377 s or 6 min 17 s
- 656 s or 10 min 57 s
- 2642 s or 44 min 2 s
2.5.4 The Carrington Event
- CME speed is 2.37 × 106 m/s or 8.5 million km/h
Media Attributions
- “Heliocentric model from Nicolaus Copernicus’ De revolutionibus orbium coelestium (On the Revolutions of the Heavenly Spheres)” by Copernicus is in the public domain.
- “January 11, 2014 NOAA jet stream wind speed map” is in the public domain.
- “Jupiter Eye to Io” by NASA is in the public domain.
- Galileo is credited as being the first to measure speed as the ratio of distance travelled to the time taken. ↵
- Average speeds and velocities are generally different than the speed and velocity at the same instant. ↵
- The other fundamental measures are: kilogram (kg) is the base measure used in quantifying mass; ampere (A) is the base measure used in quantifying electric current; Kelvin (K) is the base measure for quantifying temperature; Mole (mol) is the base measure used in quantifying the number of atoms in a substance; candela (cd) is the base measure used in quantifying the luminous intensity of any light source. ↵
- Heliacal rising refers to the moment that a star becomes briefly visible on the Eastern horizon at dawn, having not been visible during the previous year. ↵
- The sundial, while known at the same time as these two clocks, is not covered in this chapter. ↵
- [latex]\Delta d=6\text{ km}+5\text{ km}+5\text{ km}+5\text{ km}=21\text{ km}[/latex] ↵
- [latex]t=3\text{ h}+2.5\text{ h}+3\text{ h}+2\text{ h}=10.5\text{ h}[/latex] ↵
- Never heard of a killer tomato? See Attack of the Killer Tomatoes to learn more. ↵
- Galileo is credited with developing the concept of relative velocity in his early experiments, which he designed to prove the Earth was a sphere that rotated around the Sun. He published this concept in his work, “Dialogues Concerning the Two Chief World Systems”. (For more information, see "Galilean Relativity and Galileo's Ship.") ↵
- In physics, quintessence is a hypothetical form of dark energy, more precisely a scalar field, postulated as an explanation of the observation of an accelerating rate of expansion of the universe. (Wikipedia) ↵
- Venice at the time of Galileo was rather a boisterous city with a population of around 150,000 people consuming in excess of 40 million bottles of wine each year. ↵
- Galileo was the oldest son in his family and found himself responsible for raising the dowries of his younger sisters, and the financial care of three children by his mistress, Marina Gamba. ↵
- These two discoveries acted to destroy the concept of heavenly bodies made of the quintessence. Galileo’s later discovery that the Sun had spots on it further tarnished the image of the perfection of the quintessence. ↵
- Protestant churches of the time allowed for the freedom to interpret the Bible. ↵
- In 1616 the Catholic Church banned the 1543 book by Nicholas Copernicus “On the Revolutions of the Celestial Spheres,” which contained the theory that the Earth revolved around the Sun. ↵
- It was understood that Galileo’s punishment reflected his behavior towards authority and not of his scientific achievements. As written by a Jesuit scholar of his time: “If Galileo had only known how to retain the favor of the fathers of this college, he would have stood in renown before the world; he would have been spared all his misfortunes, and could have written about everything, even about the motion of the Earth.” The Jesuits were soon teaching that the Earth orbited the Sun, as in a heliocentric model in China and other parts of the world. ↵
- Mythbusters did a variation of this experiment in one of their episodes: Mythbusters - Soccer Ball Shot from Truck ↵
- Wikipedia: Astronomical Unit ↵

