Answer Key 4.2
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrr} (3)&(\dfrac{n}{3}&<&3)&\text{or}&\dfrac{-5n}{-5}&<&\dfrac{-10}{-5} \\ \\ &n&<&9&\text{or}&n&>&2 \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, \infty)[/latex]

- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrrr} \dfrac{6m}{6}&\ge & \dfrac{-24}{6}& \text{or}&m&-&7&<&-12 \\ &&&&&+&7&<&+7 \\ \hline m&\ge & -4& \text{or}&&&m&<&-5 \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -5) \cup [-4, \infty)[/latex]
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrrr} x&+&7& \ge& 12& \text{or}& \dfrac{9x}{9}&<&\dfrac{-45}{9} \\ &-&7&&-7&&&& \\ \hline &&x& \ge&5& \text{or}&x&<&-5 \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -5) \cup [5, \infty)[/latex]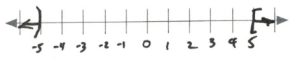
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrrr} \dfrac{10r}{10}&>&\dfrac{0}{10}&\text{or}&r&-&5&<&-12 \\ &&&&&+&5&&+5 \\ \hline r&>&0&\text{or}&&&r&<&-7 \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -7) \cup (0, \infty)[/latex]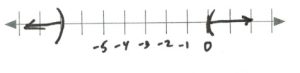
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrrr} x&-&6&<&-13&\text{or}&\dfrac{6x}{6}&<&\dfrac{-60}{6} \\ &+&6&&+6&&&& \\ \hline &&x&<&-7&&x&<&-10 \\ \\ \end{array} \\ \text{Interval notation: } (-\infty, -7)[/latex]
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrrr} 9&+&n&<&2&\text{or}&\dfrac{5n}{5}&>&\dfrac{40}{5} \\ -9&&&&-9&&&& \\ \hline &&n&<&-7&\text{or}&n&>&8 \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -7)\cup (8, \infty)[/latex]
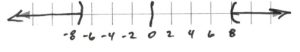
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrcrrrrr} (8)&(\dfrac{v}{8}&>&-1)&\text{and}&v&-&2&<&1 \\ &&&&&&+&2&&+2 \\ \hline &v&>&-8&\text{and}&&&v&<&3 \\ \\ &&-8&<&v&<&3&&& \\ \\ \end{array}\\ \text{Interval notation: }(-8, 3)[/latex]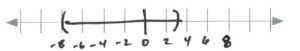
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrrr} \dfrac{-9x}{-9}&<&\dfrac{63}{-9}&\text{and}&(\dfrac{x}{4}&<&1)&(4) \\ \\ x&>&-7&\text{and}&x&<&4& \\ \\ &-7&<&x&<&4&& \\ \\ \end{array}\\ \text{Interval notation: } (-7, 4)[/latex]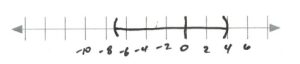
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrrr} -8&+&b&<&-3&\text{and}&\dfrac{4b}{4}&<&\dfrac{20}{4} \\ +8&&&&+8&&&& \\ \hline &&b&<&5&&b&<&5 \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, 5)[/latex]
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrrr} \dfrac{-6n}{-6}&<&\dfrac{12}{-6}&\text{and}&(\dfrac{n}{3}&<&2)&(3) \\ \\ n&>&-2&\text{and}&n&<&6& \\ \\ &-2&<&n&<&6&& \\ \\ \end{array}\\ \text{Interval notation: } (-2, 6)[/latex]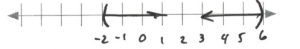
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrcrrr} a&+&10&\ge &3&\text{and}&\dfrac{8a}{8}&<&\dfrac{48}{8} \\ &-&10&&-10&&&& \\ \hline &&a&\ge &-7&\text{and}&a&<&6 \\ \\ &&&-7&\le &a&<&6&\\ \\ \end{array}\\ \text{Interval notation: } [-7, 6)[/latex]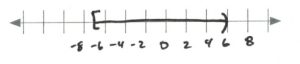
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrcrrr} -6&+&v&\ge &0&\text{and}&\dfrac{2v}{2}&>&\dfrac{4}{2} \\ +6&&&&+6&&&& \\ \hline &&v&\ge &6&\text{and}&v&>&2\\ \\ \end{array}\\ \text{Interval notation: } [6, \infty)[/latex]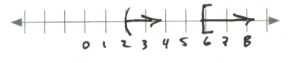
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrr} 3&<&9&+&x&<&7 \\ -9&&-9&&&&-9 \\ \hline -6&<&&x&&<&-2 \\ \\ \end{array}\\ \text{Interval notation: } (-6, -2)[/latex]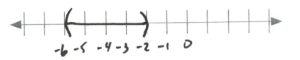
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrr} (0&\ge & \dfrac{x}{9} & \ge & -1)&(9) \\ \\ 0&\ge & x& \ge & -9& \\ \\ \end{array}\\ \text{Interval notation: } [-9, 0][/latex]
![Numberline [-9,0]](https://opentextbc.ca/intermediatealgebraberg/wp-content/uploads/sites/304/2022/11/Chapter4.2_Key14-300x60.jpg)
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrr} 11&<&8&+&k&<&12 \\ -8&&-8&&&&-8 \\ \hline 3&<&&k&&<&4 \\ \\ \end{array}\\ \text{Interval notation: } (3, 4)[/latex]
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrr} -11&<&n&-&9&<&-5 \\ +9&&&+&9&&+9 \\ \hline -2&<&&n&&<&4 \\ \\ \end{array}\\ \text{Interval notation: } (-2, 4)[/latex]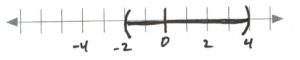
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrr} -3&<&x&-&1&<&1 \\ +1&&&+&1&&+1 \\ \hline -2&<&&x&&<&2 \\ \\ \end{array}\\ \text{Interval notation: } (-2, 2)[/latex]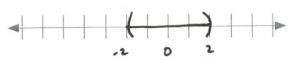
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrr} (-1&< & \dfrac{p}{8} & <& 0)&(8) \\ \\ -8&< & p& < & 0& \\ \\ \end{array}\\ \text{Interval notation: } (-8, 0)[/latex]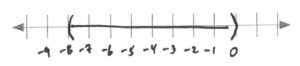
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrr} -4&<&8&-&3m&<&11 \\ -8&&-8&&&&-8 \\ \hline \dfrac{-12}{-3}&<&&\dfrac{-3m}{-3}&&<&\dfrac{3}{-3} \\ \\ 4&>&&m&&>&-1\\ \\ \end{array}\\ \text{Interval notation: } (-1, 4)[/latex]
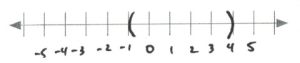
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrcrrrrr} 3&+&7r&>&59&\text{or} &-6r&-&3&>&33 \\ -3&&&&-3&&&+&3&>&+3 \\ \hline &&\dfrac{7r}{7}&>&\dfrac{56}{7}&\text{or} &&&\dfrac{-6r}{-6}&>&\dfrac{36}{-6} \\ \\ &&r&>&8&\text{or} &&&r&<&-6\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -6) \cup (8, \infty)[/latex]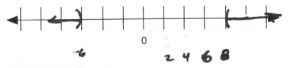
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrcrrr} -16&<&2n&-&10&<&-2 \\ +10&&&+&10&&+10 \\ \hline \dfrac{-6}{2}&<&&\dfrac{2n}{2}&&<&\dfrac{8}{2} \\ \\ -3&<&&n&&<&4 \\ \\ \end{array}\\ \text{Interval notation: } (-3, 4)[/latex]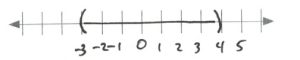
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrcrrrrr} -6&-&8x&\ge &-6&\text{or} &2&+&10x&>&82 \\ +6&&&&+6&&-2&&&>&-2 \\ \hline &&\dfrac{-8x}{-8}&\ge&\dfrac{0}{-8}&&&&\dfrac{10x}{10}&>&\dfrac{80}{10} \\ \\ &&x&\le &0&&\text{or}&&x&>&8\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, 0] \cup (8, \infty)[/latex]

- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrcrrrrr} -5b&+&10&<&30&\text{and} &7b&+&2&<&-40 \\ &&-10&&-10&&&-&2&&-2 \\ \hline &&\dfrac{-5b}{-5}&<&\dfrac{20}{-5}&&&&\dfrac{7b}{7}&<&\dfrac{-42}{7} \\ \\ &&b&>&-4&&\text{and}&&b&<&-6\\ \\ \end{array}\\ \therefore \text{No solution}[/latex]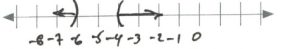
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrcrrrrr} n&+&10&\ge &15&\text{or} &4n&-&5&<&-1 \\ &-&10&&-10&&&+&5&&+5 \\ \hline &&n&\ge&5&&&&\dfrac{4n}{4}&<&\dfrac{4}{4} \\ \\ &&n&\ge &5&&\text{or}&&n&<&1\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, 1) \cup [5, \infty)[/latex]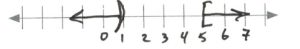
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} 3x&-&9&<&2x&+&10&\text{and}&5&+&7x&<&10x&-&10 \\ -2x&+&9&&-2x&+&9&&-5&-&10x&&-10x&-&5 \\ \hline &&x&<&19&&&&&&\dfrac{-3x}{-3}&<&\dfrac{-15}{-3}&& \\ \\ &&x&<&19&&&\text{and}&&&x&>&5&& \\ \\ &&&&&5&<&x&<&19&&&&&\\ \\ \end{array}\\ \text{Interval notation: } (5, 19)[/latex]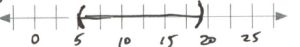
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} 4n&+&8&<&3n&-&6&\text{or}&10n&-&8&\ge &9&+&9n \\ -3n&-&8&&-3n&-&8&&-9n&+&8&&+8&-&9n \\ \hline &&n&<&-14&&&\text{or}&&&n&\ge &17&& \\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -14) \cup [17, \infty)[/latex]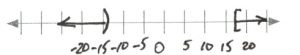
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} -8&-&6v&<&8&-&8v&\text{and}&7v&+&9&<&6&+&10v \\ +8&+&8v&&+8&+&8v&&-10v&-&9&&-9&-&10v \\ \hline &&\dfrac{2v}{2}&<&\dfrac{16}{2}&&&&&&\dfrac{-3v}{-3}&<&\dfrac{-3}{-3}&& \\ \\ &&v&<&8&&&\text{and}&&&v&>&1&& \\ \\ &&&&&1&<&v&<&8&&&&&\\ \\ \end{array}\\ \text{Interval notation: } (1, 8)[/latex]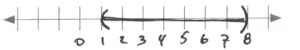
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} 5&-&2a&\ge &2a&+&1&\text{or}&10a&-&10&\ge &9a&+&9 \\ -5&-&2a&&-2a&-&5&&-9a&+&10&&-9a&+&10 \\ \hline &&\dfrac{-4a}{-4}&\ge &\dfrac{-4}{-4}&&&&&&a&\ge&19&& \\ \\ &&a&\le &1&&&\text{or}&&&a&\ge &19&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, 1] \cup [19, \infty)[/latex]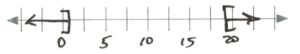
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} 1&+&5k&\ge &7k&-&3&\text{or}&k&-&10&>&2k&+&10 \\ -1&-&7k&&-7k&-&1&&-2k&+&10&&-2k&+&10 \\ \hline &&\dfrac{-2k}{-2}&\ge &\dfrac{-4}{-2}&&&&&&-k&>&20&& \\ \\ &&k&\le &2&&&\text{or}&&&k&<&-20&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, 2][/latex]![Numberline (-2, negative infinity]](https://opentextbc.ca/intermediatealgebraberg/wp-content/uploads/sites/304/2022/11/Chapter4.2_Key29-300x70.jpg)
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrl} 8&-&10r&<&8&+&4r&\text{or}&-6&+&8r&<&2&+&8r \\ -8&-&4r&&-8&-&4r&&+6&-&8r&&+6&-&8r \\ \hline &&\dfrac{-14r}{-14}&<&\dfrac{0}{-14}&&&&&&0&<&8&\leftarrow &\text{This is always true} \\ \\ &&r&>&0&&&\text{or}&&&r&\in &\mathbb{R} &&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, \infty)[/latex]

- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} 2x&+&9&\ge &10x&+&1&\text{and}&3x&-&2&<&7x&+&2 \\ -10x&-&9&&-10x&-&9&&-7x&+&2&&-7x&+&2 \\ \hline &&\dfrac{-8x}{-8}&\ge &\dfrac{-8}{-8}&&&&&&\dfrac{-4x}{-4}&<&\dfrac{4}{-4}&& \\ \\ &&x&\le &1&&&\text{and}&&&x&>&-1&& \\ \\ &&&&&-1&<&x&\le &1&&&&&\\ \\ \end{array}\\ \text{Interval notation: } (-1, 1][/latex]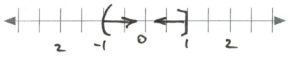
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrrcrrrrrrr} -9m&+&2&< &-10&-&6m&\text{or}&-m&+&5&\ge &10&+&4m \\ +6m&-&2&&-2&+&6m&&-4m&-&5&&-5&-&4m \\ \hline &&\dfrac{-3m}{-3}&<&\dfrac{-12}{-3}&&&&&&\dfrac{-5m}{-5}&\ge &\dfrac{5}{-5}&& \\ \\ &&m&>&4&&&\text{or}&&&m&\le &-1&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -1] \cup (4, \infty)[/latex]