Answer Key 4.3

  1. [latex]\begin{array}{rrcrrr} -3& <& x& <& 3& \hspace{0.25in} \text{Interval notation: } (-3,3) \end{array}[/latex] (-3,3)
  2. [latex]\begin{array}{rrcrrr} -8& \le& x& \le& 8 & \hspace{0.25in} \text{Interval notation: } [-8,8] \end{array}[/latex]
    Numberline (-3,3)
  3. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrcrrr} \dfrac{-6}{2}&<&\dfrac{2x}{2}&<&\dfrac{6}{2}& \\ \\ -3&<&x&<&3& \hspace{0.25in} \text{Interval notation: } (-3,3) \end{array}[/latex] Numerline (-8,8)
  4. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -4&<&x&+&3&<&4& \\ -3&&&-&3&&-3& \\ \hline -7&<&&x&&<&1& \hspace{0.25in} \\ \\ \end{array}\\ \text{Interval notation: } (-7,1)[/latex]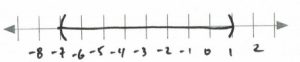 Numberline (-7,1)
  5. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -6&<&x&-&2&<&6& \\ +2&&&+&2&&+2& \\ \hline -4&<&&x&&<&8& \hspace{0.25in}\\ \\ \end{array}\\ \text{Interval notation: } (-4,8)[/latex] Numberline (-4,8)
  6. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr}-12&<&x&-&8&<&12& \\ +8&&&+&8&&+8& \\ \hline -4&<&&x&&<&20& \\ \\ \end{array}\\ \text{Interval notation: } (-4,20)[/latex]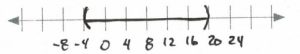 Numberline (-4, 20)
  7. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -3&<&x&-&7&<&3& \\ +7&&&+&7&&+7& \\ \hline 4&<&&x&&<&10& \\ \\ \end{array}\\ \text{Interval notation: } (4,10)[/latex]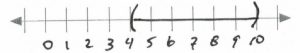 Numberline (4,10)
  8. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -4&\le &x&+&3&\le &4& \\ -3&&&-&3&&-3& \\ \hline -7&\le &&x&&\le &1& \\ \\ \end{array}\\ \text{Interval notation: } [-7,1][/latex]
    Numberline (-9, 1)
  9. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -9&<&3x&-&2&<&9& \\ +2&&&+&2&&+2& \\ \hline \dfrac{-7}{3}&<&&\dfrac{3x}{3}&&<&\dfrac{11}{3}&\\ \\ -\dfrac{7}{3}&<&&x&&<&\dfrac{11}{3}& \\ \\ \end{array}\\ \text{Interval notation: } (-\dfrac{7}{3},\dfrac{11}{3})[/latex] Numberline (-2.25, 3.75)
  10. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -9&<&2x&+&5&<&9& \\ -5&&&-&5&&-5& \\ \hline \dfrac{-14}{2}&<&&\dfrac{2x}{2}&&<&\dfrac{4}{2}&\\ \\ -7&<&&x&&<&2& \\ \\ \end{array}\\ \text{Interval notation: } (-7,2)[/latex]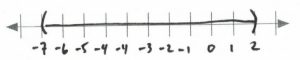 Numberline (-7,2)
  11. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} 1&+&2|x&-&1|&\le & 9 \\ -1&&&&&&-1 \\ \hline &&\dfrac{2}{2}|x&-&1|&\le & \dfrac{8}{2} \\ \\ &&|x&-&1|&\le & 4 \\ -4&\le &x&-&1&\le & 4 \\ +1&&&+&1&\le &+1 \\ \hline -3&\le &&x&&\le &5& \\ \\ \end{array}\\ \text{Interval notation: } [-3,5][/latex]
    Numberline (-3,5)
  12. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} 10&-&3|x&-&2|&\ge & 4 \\ -10&&&&&&-10 \\ \hline &&\dfrac{-3}{-3}|x&-&2|&\ge & \dfrac{-6}{-3} \\ \\ &&|x&-&2|&\le & 2 \\ -2&\le &x&-&2&\le & 2 \\ +2&&&+&2&&+2 \\ \hline 0&\le &&x&&\le &4& \\ \\ \end{array}\\ \text{Interval notation: } [0,4][/latex]
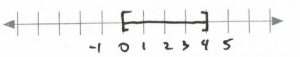
    Numberline (0,4)
  13. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrl} 6&-&|2x&-&5|&>&3& \\ -6&&&&&&-6& \\ \hline &&(-|2x&-&5|&>&-3)&(-1) \\ &&|2x&-&5|&<&3& \\ -3&<&2x&-&5&<&3& \\ +5&&&+&5&&+5& \\ \hline \dfrac{2}{2}&<&&\dfrac{2x}{2}&&<&\dfrac{8}{2}& \\ \\ 1&<&&x&&<&4& \\ \\ \end{array} \\ \text{Interval notation: } (1,4)[/latex]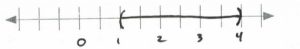 Numberline (1,4)
  14. [latex]\begin{array}{rrcrrrrrr} x& <&-5&\text{or}&5&<&x& \hspace{0.25in} \text{Interval notation: } (-\infty,-5)\cup (5, \infty) \end{array}[/latex] Numberline (- infinity, -5) or (5, infinity)
  15. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrr} \dfrac{3x}{3}&<&\dfrac{-5}{3}&\text{or}&\dfrac{5}{3}&<&\dfrac{3x}{3}& \\ \\ x&<&-\dfrac{5}{3}&\text{or}&\dfrac{5}{3}&<&x&\\ \\ \end{array} \\ \text{Interval notation: } (-\infty, -\dfrac{5}{3})\cup (\dfrac{5}{3}, \infty) \\[/latex]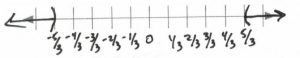 (- infinity, -5/3) or (5/3, infinity)
  16. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrrr} x&-&4&<&-5&\text{or}&5&<&x&-&4&\\ &+&4&&+4&\text{or}&+4&&&+&4&\\ \hline &&x&<&-1&\text{or}&9&<&x&&&\\ \\ \end{array} \\ \text{Interval notation: } (-\infty, -1)\cup (9, \infty)[/latex]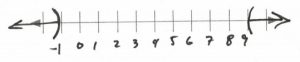 (- infinity, -1) or (9, infinity)
  17. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrrr} x&+&3&<&-3&\text{or}&3&<&x&+&3& \\ &-&3&&-3&\text{or}&-3&&&-&3& \\ \hline &&x&<&-6&\text{or}&0&<&x&&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -6)\cup (0, \infty)\\[/latex] (- infinity, -6) or (0, infinity)
  18. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrrr} 2x&-&4&<&-6&\text{or}&6&<&2x&-&4& \\ &+&4&&+4&&+4&&&+&4& \\ \hline &&\dfrac{2x}{2}&<&\dfrac{-2}{2}&&\dfrac{10}{2}&<&\dfrac{2x}{2}&&& \\ \\ &&x&<&-1&\text{or}&5&<&x&&&\\ \\ \end{array} \\ \text{Interval notation: } (-\infty, -1)\cup (5, \infty)\\[/latex]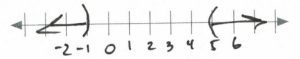 (negative infinity, -1) or (5, infinity)
  19. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrrr} x&-&5&<&-3&\text{or}&3&<&x&-&5& \\ &+&5&&+5&&+5&&&+&5& \\ \hline &&x&<&2&\text{or}&8&<&x&&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, 2)\cup (8, \infty)\\[/latex] HAVE TO ADD 2 2 etc.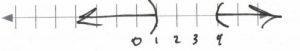 (1, negative infinity) or (4, infinity)
  20. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrl} 3&-|2&-&x|&<&1&&&&&\\ -3&&&&&-3&&&&&\\ \hline &(-|2&-&x|&<&-2)&(-1)&&&&\\ &|2&-&x|&>&2&&&&&\\ \\ 2&-&x&<&-2&\text{or}&2&<&2&-&x\\ -2&&&&-2&&-2&&-2&&\\ \hline &&-x&<&-4&\text{or}&0&<&-x&&\\ &&x&>&4&\text{or}&0&>&x&&\\ \\ \end{array} \\ \text{Interval notation: } (-\infty, 0)\cup (4, \infty)\\[/latex]
    (negative infinty, 0) or (4, infinity)
  21. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrl} 4&+&3|x&-&1|&<&10&&&&\\ -4&&&&&&-4&&&&\\ \hline &&\dfrac{3}{3}|x&-&1|&<&\dfrac{6}{3}&&&&\\ \\ &&|x&-&1|&<&2&&&&\\ \\ x&-&1&<&-2&\text{or}&2&<&x&-&1\\ &+&1&&+1&&+1&&&+&1\\ \hline &&x&<&-1&\text{or}&3&<&x&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -1)\cup (3, \infty)\\[/latex]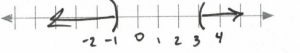 (negative infinity, -1) or (3, infinity)
  22. [latex]\phantom{a}[/latex]
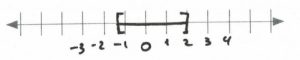
    [latex]\begin{array}[t]{rrrcrrrl} 3&-&2|3x&-&1|&\ge &-7& \\ -3&&&&&&-3& \\ \hline &&\dfrac{-2}{-2}|3x&-&1|&\ge &\dfrac{-10}{-2}& \\ \\ &&|3x&-&1|&\le &5& \\ \\ \\ -5&\le &3x&-&1& \le & 5& \\ +1&&&+&1&&+1& \\ \hline \dfrac{-4}{3}&\le &&\dfrac{3x}{3}&& \le & \dfrac{6}{3}& \\ \\ -\dfrac{4}{3}&\le &&x&& \le & 2 &\\ \\ \end{array}\\ \text{Interval notation: } [-\dfrac{4}{3}, 2][/latex]
    (-4/3, 2)
  23. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrcrrrrl} 3&-&2|x&-&5|&\le & -15&&&& \\ -3&&&&&&-3&&&& \\ \hline &&\dfrac{-2}{-2}|x&-&5|&\le & \dfrac{-18}{-2}&&&& \\ \\ &&|x&-&5|&\ge & 9&&&& \\ \\ x&-&5&\le &-9&\text{or}&9&\le &x&-&5 \\ &+&5&&+5&&+5&&&+&5 \\ \hline &&x&\le &-4&\text{or}&14&\le &x&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -4]\cup [14, \infty)[/latex]
    (- ifninity, -4] or [14, infinity)
  24. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrcrrcrrrll} 4&-&6|-6&-&3x|&\le & -5&&&& \\ -4&&&&&&-4&&&& \\ \hline &&\dfrac{-6}{-6}|-6&-&3x|&\le &\dfrac{-9}{-6}&&&& \\ \\ &&|-6&-&3x|&\ge &\dfrac{3}{2}&&&& \\ \\ \\ -6&-&3x&\le &-\dfrac{3}{2}&\text{or}&\dfrac{3}{2}&\le &-6&-\phantom{0}3x& \\ \\ +6&&&&+6&&+6&&+6&& \\ \hline &&\dfrac{-3x}{-3}&\le &\dfrac{\dfrac{9}{2}}{-3}&&\dfrac{\dfrac{15}{2}}{-3}&\le &\dfrac{-3x}{-3}&& \\ \\ &&x&\ge &-\dfrac{3}{2}&\text{or}&-\dfrac{5}{2}&\ge &x&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -\dfrac{5}{2}]\cup [-\dfrac{3}{2}, \infty)[/latex]
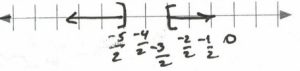
    [-5/2, negative infinty) or [-2/3, infinity)
  25. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrr} -2&-&3|4&-&2x|&\ge & -8& \\ +2&&&&&&+2& \\ \hline &&\dfrac{-3}{-3}|4&-&2x|&\ge &\dfrac{-6}{-3}& \\ \\ &&|4&-&2x|&\le &2& \\ \\ \\ -2&\le &4&-&2x&\le &2& \\ -4&&-4&&&&-4& \\ \hline \dfrac{-6}{-2}&\le &&\dfrac{-2x}{-2}&&\le &\dfrac{-2}{-2}& \\ \\ 3&\ge &&x&&\ge &1&\\ \\ \end{array}\\ \text{Interval notation: } [1,3][/latex]
    [1,3]
  26. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrcrr} -3&-&2|4x&-&5|&\ge & 1& \\ +3&&&&&&+3& \\ \hline &&\dfrac{-2}{-2}|4x&-&5|&\ge &\dfrac{4}{-2}& \\ \\ &&|4x&-&5|&\le &-2& \\ \\ &&&&&\uparrow&& \\ &&&&&\text{Cannot be true.}&&\text{No solution.} \end{array}[/latex]
    Numerline no solution
  27. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrrl} 4&-&5|-2x&-&7|&<&-1&&&&& \\ -4&&&&&&-4&&&&& \\ \hline &&\dfrac{-5}{-5}|-2x&-&7|&<&\dfrac{-5}{-5}&&&&& \\ \\ &&|-2x&-&7|&>&1&&&&& \\ \\ \\ -2x&-&7&<&-1&\text{or}&1&<&-2x&-&7& \\ &+&7&&+7&&+7&&&+&7& \\ \hline &&\dfrac{-2x}{-2}&<&\dfrac{6}{-2}&&\dfrac{8}{-2}&<&\dfrac{-2x}{-2}&&& \\ \\ &&x&>&-3&\text{or}&-4&>&x&&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -4)\cup (-3, \infty)[/latex]
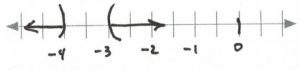
    (negative infinity, -4) or (-3, infinity)
  28. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrl} -2&+&3|5&-&x|& \le& 4& \\ +2&&&&&&+2& \\ \hline &&\dfrac{3}{3}|5&-&x|& \le&\dfrac{6}{3}& \\ \\ &&|5&-&x|& \le&2& \\ \\ -2&\le &5&-&x&\le &2& \\ -5&&-5&&&&-5& \\ \hline (-7&\le &&-x&&\le &-3)&(-1) \\ 7&\ge &&x&&\ge &3&\\ \\ \end{array} \\ \text{Interval notation: } [3,7][/latex]
    [3,7]
  29. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrl} 3&-&2|4x&-&5|&\ge &1& \\ -3&&&&&&-3& \\ \hline &&\dfrac{-2}{-2}|4x&-&5|&\ge &\dfrac{-2}{-2}& \\ \\ &&|4x&-&5|&\le &1& \\ \\ -1&\le &4x&-&5&\le &1& \\ +5&&&+&5&&+5& \\ \hline \dfrac{4}{4}&\le &&\dfrac{4x}{4}&&\le &\dfrac{6}{4}& \\ \\ 1&\le &&x&&\le &\dfrac{3}{2}&\\ \\ \end{array} \\ \text{Interval notation: } [1, \dfrac{3}{2}][/latex]
    [2/2, 3/2]
  30. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrl} -2&-&3|-3x&-&5|&\ge &-5& \\ +2&&&&&&+2& \\ \hline &&\dfrac{-3}{-3}|-3x&-&5|&\ge &\dfrac{-3}{-3}& \\ \\ &&|-3x&-&5|&\le &1& \\ \\ -1&\le &-3x&-&5&\le &1& \\ +5&&&+&5&&+5& \\ \hline \dfrac{4}{-3}&\le &&\dfrac{-3x}{-3}&&\le &\dfrac{6}{-3}& \\ \\ -\dfrac{4}{3}&\ge &&x&&\ge &-2&\\ \\ \end{array}\\ \text{Interval notation: } [-2, -\dfrac{4}{3}][/latex]
    [-6/3, -4/3]
  31. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrl} -5&-&2|3x&-&6|&<&-8&&&& \\ +5&&&&&&+5&&&& \\ \hline &&\dfrac{-2}{-2}|3x&-&6|&<&\dfrac{-3}{-2}&&&& \\ \\ &&|3x&-&6|&>&\dfrac{3}{2}&&&& \\ \\ \\ 3x&-&6&<&-\dfrac{3}{2}&\text{or}&\dfrac{3}{2}&<&3x&-&6 \\ \\ &+&6&&+6&&+6&&&+&6 \\ \hline &&\dfrac{3x}{3}&<&\dfrac{\dfrac{9}{2}}{3}&&\dfrac{\dfrac{15}{2}}{3}&<&\dfrac{3x}{3}&& \\ \\ &&x&<&\dfrac{3}{2}&\text{or}&5&<&x&&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, \dfrac{3}{2})\cup (5, \infty)[/latex]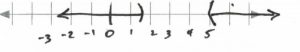 (negative infinity, -1/2) or (5, infinity)
  32. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrrrrrrrrrl} 6&-&3|1&-&4x|&<&-3&&&&& \\ -6&&&&&&-6&&&&& \\ \hline &&\dfrac{-3}{-3}|1&-&4x|&<&\dfrac{-9}{-3}&&&&& \\ \\ &&|1&-&4x|&>&3&&&&& \\ \\ 1&-&4x&<&-3&\text{or}&1&-&4x&>&3& \\ -1&&&&-1&&-1&&&&-1& \\ \hline &&-4x&<&-4&&&&-4x&>&2& \\ &&x&>&1&&\text{or}&&x&<&-\dfrac{1}{2}&\\ \\ \end{array}\\ \text{Interval notation: } (-\infty, -\dfrac{1}{2})\cup (1, \infty)[/latex]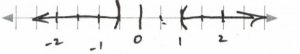 (negative infinity, -1/2) or (1, infinity)
  33. [latex]\phantom{a}[/latex]
    [latex]\begin{array}[t]{rrrcrrrl} 4&-&4|-2x&+&6|&>&-4& \\ -4&&&&&&-4& \\ \hline &&\dfrac{-4}{-4}|-2x&+&6|&>&\dfrac{-8}{-4}& \\ \\ &&|-2x&+&6|&<&2& \\ \\ -2&<&-2x&+&6&<&2& \\ -6&&&-&6&&-6& \\ \hline \dfrac{-8}{-2}&<&&\dfrac{-2x}{-2}&&<&\dfrac{-4}{-2}& \\ \\ 4&>&&x&&>&2&\\ \\ \end{array}\\ \text{Interval notation: } (2,4)[/latex]
    (2,4)

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Intermediate Algebra Copyright © 2020 by Terrance Berg is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book