Midterm 1: Version E Answer Key
-
- −9
- 9
- −9
- 10
- −3
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrlrrrr} 2x&-&8&+&18&=&-12&+&4x&+&12 \\ -2x&&&&&&&-&2x&& \\ \hline &&&&\dfrac{10}{2}&=&\dfrac{2x}{2}&&&& \\ \\ &&&&x&=&5&&&& \end{array}[/latex] - [latex]\left(\dfrac{1}{R}-\dfrac{1}{r_1}=\dfrac{1}{r_2}\right)(Rr_1r_2)[/latex]
[latex]\begin{array}{rrrrlrr} r_1r_2&-&Rr_2&=&\phantom{-}Rr_1&& \\ -Rr_1&+&Rr_2&&-Rr_1&+&Rr_2 \\ \hline r_1r_2&-&Rr_1&=&Rr_2&& \\ r_1(r_2&-&R)&=&Rr_2&& \\ \\ &&r_1&=&\dfrac{Rr_2}{r_2-R}&& \end{array}[/latex] - [latex]\left(\dfrac{x}{12}-\dfrac{x-4}{3}=\dfrac{2}{3} \right)(12)[/latex]
[latex]\begin{array}{rrcrrrl} x&-&4(x&-&4)&=&4(2) \\ x&-&4x&+&16&=&8 \\ &&&-&16&&-16 \\ \hline &&&&\dfrac{-3x}{-3}&=&\dfrac{-8}{-3} \\ \\ &&&&x&=&\dfrac{8}{3} \end{array}[/latex] - [latex]y=-6[/latex]
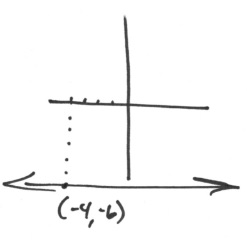
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{ll} \begin{array}[t]{rrl} m&=&\dfrac{\Delta y}{\Delta x} \\ \\ \dfrac{2}{5}&=&\dfrac{y-1}{x--1} \end{array} & \hspace{0.25in} \begin{array}[t]{rrrrrrlrr} &&2(x&+&1)&=&\phantom{-}5(y&-&1) \\ &&2x&+&2&=&\phantom{-}5y&-&5 \\ &-&5y&+&5&&-5y&+&5 \\ \hline 2x&-&5y&+&7&=&0&& \\ \\ &&&&y&=&\dfrac{2}{5}x&+&\dfrac{7}{5} \end{array} \end{array}[/latex] - [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{ll} \begin{array}[t]{rrl} &&\textbf{1st slope:} \\ \\ m&=&\dfrac{\Delta y}{\Delta x} \\ \\ m&=&\dfrac{5--1}{2-0} \\ \\ m&=&\dfrac{6}{2} \\ \\ m&=&3 \end{array} & \hspace{0.25in} \begin{array}[t]{rrl} &&\textbf{Now:} \\ \\ m&=&\dfrac{\Delta y}{\Delta x} \\ \\ 3&=&\dfrac{y--1}{x-0} \\ \\ 3x&=&y+1 \\ \\ 3x-y-1&=&0 \\ \\ \therefore 0&=&3x-y-1 \\ y&=&3x-1 \end{array} \end{array}[/latex] - [latex]\phantom{a}[/latex]
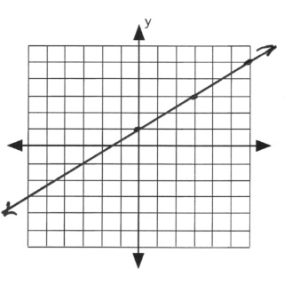
- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{rrrrrrr} -20&\le &8x&-&4&\le &28 \\ +4&&&+&4&&+4 \\ \hline \dfrac{-16}{8}&\le &&\dfrac{8x}{8}&&\le &\dfrac{32}{8} \\ \\ -2&\le &&x&&\le &4 \end{array}[/latex]
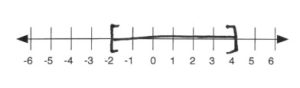
- [latex]\left(-2\le \dfrac{2x+2}{6} \le 2 \right)(6)[/latex]
[latex]\begin{array}{rrrrrrr} -12&\le &2x&+&2&\le &12 \\ -2&&&-&2&&-2 \\ \hline \dfrac{-14}{2}&\le &&\dfrac{2x}{2}&&\le &\dfrac{10}{2} \\ \\ -7&\le &&x&&\le &5 \end{array}[/latex]

- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{ll} \left(\dfrac{3x-4}{5} < -1 \right)(5) \hspace{0.25in}& \text{or} \hspace{0.25in} \left(1 < \dfrac{3x-4}{5}\right)(5) \\ \\ \begin{array}[t]{rrrrr} 3x&-&4&<&-5 \\ &+&4&&+4 \\ \hline &&3x&<&-1 \\ \\ &&x&<&-\dfrac{1}{3} \end{array} & \hspace{0.25in} \begin{array}[t]{rrrrr} 5&<&3x&-&4 \\ +4&&&+&4 \\ \hline \dfrac{9}{3}&<&\dfrac{3x}{3}&& \\ \\ x&>&3&& \end{array} \end{array}[/latex]
 -1 over 3, x < 3" width="300" height="71">
-1 over 3, x < 3" width="300" height="71"> -
[latex]3x-2y<12[/latex] [latex]x[/latex] [latex]y[/latex] 0 −6 4 0 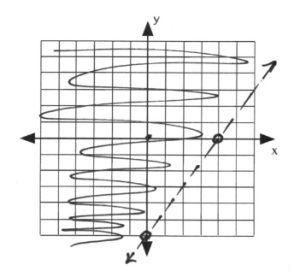
- [latex]x, x+2, x+4[/latex]
[latex]\begin{array}[t]{rrcrrrcrrrl} x&+&2(x&+&2)&+&3(x&+&4)&=&\phantom{-}94 \\ x&+&2x&+&4&+&3x&+&12&=&\phantom{-}94 \\ &&&&&&6x&+&16&=&\phantom{-}94 \\ &&&&&&&-&16&&-16 \\ \hline &&&&&&&&\dfrac{6x}{6}&=&\dfrac{78}{6} \\ \\ &&&&&&&&x&=&13\\ \end{array}[/latex]
numbers are 13, 15, 17 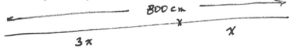
[latex]\begin{array}[t]{rrl} 3x+x&=&800\text{ cm} \\ 4x&=&800\text{ cm} \\ x&=&\dfrac{800\text{ cm}}{4}\text{ or }200\text{ cm} \\ \\ 3x&=&600\text{ cm} \end{array}[/latex]- [latex]\phantom{a}[/latex]
[latex]\begin{array}[t]{ll} \begin{array}[t]{rrl} y&=&\dfrac{km}{n^2} \\ \\ &&\underline{\text{1st}} \\ y&=&12 \\ k&=&\text{find} \\ m&=&3 \\ n&=&4 \\ \\ 12&=&\dfrac{k(3)}{(4)^2} \\ \\ k&=&\dfrac{12\cdot (4)^2}{3} \\ \\ k&=&64 \end{array} & \hspace{0.25in} \begin{array}[t]{rrl} &&\underline{\text{2nd}} \\ y&=&\text{find} \\ k&=&64 \\ m&=&3 \\ n&=&-3 \\ \\ y&=&\dfrac{(64)(3)}{(-3)^2} \\ \\ y&=&\dfrac{64}{3} \end{array} \end{array}[/latex]

