CHAPTER 8 Polynomials
8.5 Factor Quadratic Trinomials with Leading Coefficient 1
Learning Objectives
By the end of this section, you will be able to:
- Factor trinomials of the form
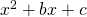
- Factor trinomials of the form
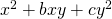
Factor Trinomials of the Form x2 + bx + c
You have already learned how to multiply binomials using FOIL. Now you’ll need to “undo” this multiplication—to start with the product and end up with the factors. Let’s look at an example of multiplying binomials to refresh your memory.
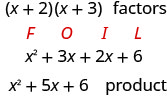
To factor the trinomial means to start with the product, ![]() , and end with the factors,
, and end with the factors, ![]() . You need to think about where each of the terms in the trinomial came from.
. You need to think about where each of the terms in the trinomial came from.
The first term came from multiplying the first term in each binomial. So to get ![]() in the product, each binomial must start with an x.
in the product, each binomial must start with an x.
The last term in the trinomial came from multiplying the last term in each binomial. So the last terms must multiply to 6
What two numbers multiply to 6?
The factors of 6 could be 1 and 6, or 2 and 3. How do you know which pair to use?
Consider the middle term. It came from adding the outer and inner terms.
So the numbers that must have a product of 6 will need a sum of 5. We’ll test both possibilities and summarize the results in the table below—the table will be very helpful when you work with numbers that can be factored in many different ways.
| Factors of |
Sum of factors |
|---|---|
We see that 2 and 3 are the numbers that multiply to 6 and add to 5. So we have the factors of ![]() . They are
. They are ![]() .
.
You should check this by multiplying.
Looking back, we started with ![]() , which is of the form
, which is of the form ![]() , where
, where ![]() and
and ![]() . We factored it into two binomials of the form
. We factored it into two binomials of the form ![]() and
and ![]() .
.
To get the correct factors, we found two numbers m and n whose product is c and sum is b.
EXAMPLE 1
Factor: ![]() .
.




TRY IT 1.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 1.2
Factor: ![]() .
.
Show answer
![]()
Let’s summarize the steps we used to find the factors.
HOW TO:
Factor trinomials of the form ![]() .
.
- Write the factors as two binomials with first terms x:
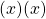 .
. - Find two numbers m and n that
Multiply to c,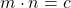
Add to b,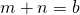
- Use m and n as the last terms of the factors:
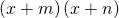 .
. - Check by multiplying the factors.
EXAMPLE 2
Factor: ![]() .
.
Notice that the variable is u, so the factors will have first terms u.
![]()
Find two numbers that: multiply to 24 and add to 11
| Factors of |
Sum of factors |
|---|---|
Use 3 and 8 as the last terms of the binomials. ![]()
Check.
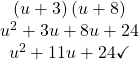
TRY IT 2.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 2.2
Factor: ![]() .
.
Show answer
![]()
EXAMPLE 3
Factor: ![]() .
.
![]()
Find two numbers that multiply to 60 and add to 17
| Factors of |
Sum of factors |
|---|---|
Use 5 and 12 as the last terms. ![]()
Check.
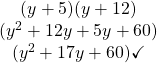
TRY IT 3.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 3.2
Factor: ![]() .
.
Show answer
![]()
Factor Trinomials of the Form x2 + bx + c with b Negative, c Positive
In the examples so far, all terms in the trinomial were positive. What happens when there are negative terms? Well, it depends which term is negative. Let’s look first at trinomials with only the middle term negative.
Remember: To get a negative sum and a positive product, the numbers must both be negative.
Again, think about FOIL and where each term in the trinomial came from. Just as before,
- the first term,
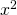 , comes from the product of the two first terms in each binomial factor, x and y;
, comes from the product of the two first terms in each binomial factor, x and y; - the positive last term is the product of the two last terms
- the negative middle term is the sum of the outer and inner terms.
How do you get a positive product and a negative sum? With two negative numbers.
EXAMPLE 4
Factor: ![]() .
.
Again, with the positive last term, 28, and the negative middle term, ![]() , we need two negative factors. Find two numbers that multiply 28 and add to
, we need two negative factors. Find two numbers that multiply 28 and add to ![]() .
.
![]()
Find two numbers that: multiply to 28 and add to ![]() .
.
| Factors of |
Sum of factors |
|---|---|
Use -4, -7 as the last terms of the binomials. ![]()
Check.
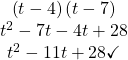
TRY IT 4.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 4.2
Factor: ![]() .
.
Show answer
![]()
Factor Trinomials of the Form x2 + bx + c with c Negative
Now, what if the last term in the trinomial is negative? Think about FOIL. The last term is the product of the last terms in the two binomials. A negative product results from multiplying two numbers with opposite signs. You have to be very careful to choose factors to make sure you get the correct sign for the middle term, too.
Remember: To get a negative product, the numbers must have different signs.
EXAMPLE 5
Factor: ![]() .
.
To get a negative last term, multiply one positive and one negative. We need factors of ![]() that add to positive 4
that add to positive 4
| Factors of |
Sum of factors |
|---|---|
Notice: We listed both ![]() and
and ![]() to make sure we got the sign of the middle term correct.
to make sure we got the sign of the middle term correct.

Check.
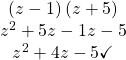
TRY IT 5.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 5.2
Factor: ![]() .
.
Show answer
![]()
Let’s make a minor change to the last trinomial and see what effect it has on the factors.
EXAMPLE 6
Factor: ![]() .
.
This time, we need factors of ![]() that add to
that add to ![]() .
.
| Factors of |
Sum of factors |
|---|---|

Check.

Notice that the factors of ![]() are very similar to the factors of
are very similar to the factors of ![]() . It is very important to make sure you choose the factor pair that results in the correct sign of the middle term.
. It is very important to make sure you choose the factor pair that results in the correct sign of the middle term.
TRY IT 6.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 6.2
Factor: ![]() .
.
Show answer
![]()
EXAMPLE 7
Factor: ![]() .
.

| Factors of |
Sum of factors |
|---|---|
Check.
![]()
![]()
![]()
TRY IT 7.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 7.1
Factor: ![]() .
.
Show answer
![]()
Some trinomials are prime. The only way to be certain a trinomial is prime is to list all the possibilities and show that none of them work.
EXAMPLE 8
Factor: ![]() .
.
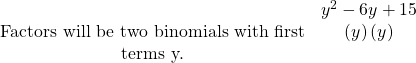
| Factors of 15 | Sum of factors |
|---|---|
As shown in the table, none of the factors add to ![]() ; therefore, the expression is prime.
; therefore, the expression is prime.
TRY IT 8.1
Factor: ![]() .
.
Show answer
prime
TRY IT 8.2
Factor: ![]() .
.
Show answer
prime
EXAMPLE 9
Factor: ![]() .
.
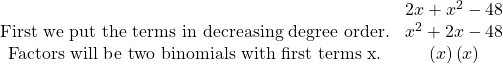
As shown in the table, you can use ![]() as the last terms of the binomials.
as the last terms of the binomials.
| Factors of |
Sum of factors |
|---|---|
Check.
![]()
![]()
![]()
TRY IT 9.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 9.2
Factor: ![]() .
.
Show answer
![]()
Let’s summarize the method we just developed to factor trinomials of the form ![]() .
.
HOW TO:
Factor trinomials of the form ![]() .
.
When we factor a trinomial, we look at the signs of its terms first to determine the signs of the binomial factors.
When c is positive, m and n have the same sign.
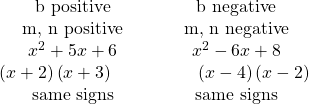
When c is negative, m and n have opposite signs.
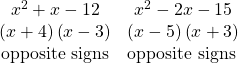
Notice that, in the case when m and n have opposite signs, the sign of the one with the larger absolute value matches the sign of b.
Factor Trinomials of the Form x2 + bxy + cy2
Sometimes you’ll need to factor trinomials of the form ![]() with two variables, such as
with two variables, such as ![]() . The first term,
. The first term, ![]() , is the product of the first terms of the binomial factors,
, is the product of the first terms of the binomial factors, ![]() . The
. The ![]() in the last term means that the second terms of the binomial factors must each contain y. To get the coefficients b and c, you use the same process summarized in the previous objective.
in the last term means that the second terms of the binomial factors must each contain y. To get the coefficients b and c, you use the same process summarized in the previous objective.
EXAMPLE 10
Factor: ![]() .
.
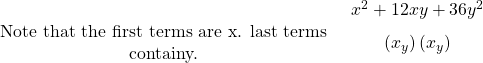
Find the numbers that multiply to 36 and add to 12
| Factors of |
Sum of factors |
|---|---|
| 1, 36 | |
| 2, 18 | |
| 3, 12 | |
| 4, 9 | |
| 6, 6 |
Use 6 and 6 as the coefficients of the last terms. ![]()
Check your answer.
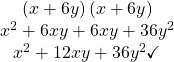
TRY IT 10.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 10.2
Factor: ![]() .
.
Show answer
![]()
EXAMPLE 11
Factor: ![]() .
.
We need ![]() in the first term of each binomial and
in the first term of each binomial and ![]() in the second term. The last term of the trinomial is negative, so the factors must have opposite signs.
in the second term. The last term of the trinomial is negative, so the factors must have opposite signs.
![]()
Find the numbers that multiply to ![]() and add to
and add to ![]() .
.
| Factors of |
Sum of factors |
|---|---|
Check your answer.Use 1, -9 as coefficients of the last terms. ![]()
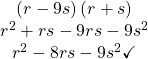
TRY IT 11.1
Factor: ![]() .
.
Show answer
![]()
TRY IT 11.2
Factor: ![]() .
.
Show answer
![]()
EXAMPLE 12
Factor: ![]() .
.
We need u in the first term of each binomial and ![]() in the second term. The last term of the trinomial is negative, so the factors must have opposite signs.
in the second term. The last term of the trinomial is negative, so the factors must have opposite signs.
![]()
Find the numbers that multiply to ![]() and add to
and add to ![]() .
.
| Factors of |
Sum of factors |
|---|---|
Note there are no factor pairs that give us ![]() as a sum. The trinomial is prime.
as a sum. The trinomial is prime.
TRY IT 12.1
Factor: ![]() .
.
Show answer
prime
TRY IT 12.2
Factor: ![]() .
.
Show answer
prime
Key Concepts
- Factor trinomials of the form
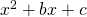
- Write the factors as two binomials with first terms x:
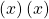 .
. - Find two numbers m and n that
Multiply to c,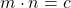
Add to b,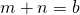
- Use m and n as the last terms of the factors:
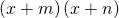 .
. - Check by multiplying the factors.
- Write the factors as two binomials with first terms x:
Practice Makes Perfect
Factor Trinomials of the Form 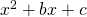
In the following exercises, factor each trinomial of the form ![]() .
.
| 1. |
2. |
| 3. |
4. |
| 5. |
6. |
| 7. |
8. |
| 9. |
10. |
| 11. |
12. |
| 13. |
14. |
| 15. |
16. |
| 17. |
18. |
| 19. |
20. |
| 21. |
22. |
| 23. |
24. |
| 25. |
26. |
| 27. |
28. |
| 29. |
30. |
| 31. |
32. |
| 33. |
34. |
Factor Trinomials of the Form 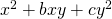
In the following exercises, factor each trinomial of the form ![]() .
.
| 35. |
36. |
| 37. |
38. |
| 39. |
40. |
| 41. |
42. |
| 43. |
44. |
| 45. |
46. |
| 47. |
48. |
| 49. |
50. |
Mixed Practice
In the following exercises, factor each expression.
| 51. |
52. |
| 53. |
54. |
| 55. |
56. |
| 57. |
58. |
| 59. |
60. |
| 61. |
62. |
| 63. |
64. |
| 65. |
66. |
Everyday Math
| 67. Consecutive integers Deirdre is thinking of two consecutive integers whose product is 56. The trinomial |
68. Consecutive integers Deshawn is thinking of two consecutive integers whose product is 182. The trinomial |
Writing Exercises
| 69. Many trinomials of the form |
70. How do you determine whether to use plus or minus signs in the binomial factors of a trinomial of the form |
| 71. Will factored |
72. Look at (Figure), where we factored |
Answers
| 1. |
3. |
| 5. |
7. |
| 9. |
11. |
| 13. |
15. |
| 17. |
19. |
| 21. |
23. |
| 25. |
27. |
| 29. prime | 31. |
| 33. |
35. |
| 37. |
39. |
| 41. |
43. |
| 45. |
47. prime |
| 49. prime | 51. |
| 53. |
55. |
| 57. |
59. prime |
| 61. |
63. |
| 65. prime | 67. |
| 69. Answers may vary | 71. Answers may vary |
Attributions
This chapter has been adapted from “Factor Trinomials of the Form ![]() ” in Elementary Algebra (OpenStax) by Lynn Marecek and MaryAnne Anthony-Smith, which is under a CC BY 4.0 Licence. Adapted by Izabela Mazur. See the Copyright page for more information.
” in Elementary Algebra (OpenStax) by Lynn Marecek and MaryAnne Anthony-Smith, which is under a CC BY 4.0 Licence. Adapted by Izabela Mazur. See the Copyright page for more information.

