CHAPTER 4 Ratio, Proportion, and Percent
4.4 Solve General Applications of Percent
Learning Objectives
By the end of this section, you will be able to:
- Translate and solve basic percent equations
- Solve applications of percent
- Find percent increase and percent decrease
Translate and Solve Basic Percent Equations
In the last section, we solved percent problems by setting them up as proportions. That is the best method available when you did not have the tools of algebra. Now, in this section we will translate word sentences into algebraic equations, and then solve the percent equations.
We’ll look at a common application of percent—tips to a server at a restaurant—to see how to set up a basic percent application.
When Kim and her friends went on a road trip to Vancouver, they ate lunch at Marta’s Cafe Tower. The bill came to ![]() . They wanted to leave a
. They wanted to leave a ![]() tip. What amount would the tip be?
tip. What amount would the tip be?
To solve this, we want to find what amount is ![]() of
of ![]() . The
. The ![]() is called the base. The amount of the tip would be
is called the base. The amount of the tip would be ![]() , or
, or ![]() See (Figure 1). To find the amount of the tip, we multiplied the percent by the base.
See (Figure 1). To find the amount of the tip, we multiplied the percent by the base.
A ![]() tip for an
tip for an ![]() restaurant bill comes out to
restaurant bill comes out to ![]() .
.

In the next examples, we will find the amount. We must be sure to change the given percent to a decimal when we translate the words into an equation.
EXAMPLE 1
What number is ![]() of
of ![]()
| Translate into algebra. Let |
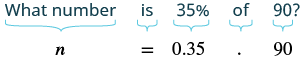 |
| Multiply. | 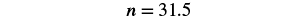 |
TRY IT 1.1
What number is ![]() of
of ![]()
Show answer
36
TRY IT 1.2
What number is ![]() of
of ![]()
Show answer
33
EXAMPLE 2
![]() of
of ![]() is what number?
is what number?
| Translate into algebra. Let |
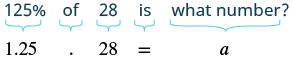 |
| Multiply. |  |
Remember that a percent over ![]() is a number greater than
is a number greater than ![]() . We found that
. We found that ![]() of
of ![]() is
is ![]() , which is greater than
, which is greater than ![]() .
.
TRY IT 2.1
![]() of
of ![]() is what number?
is what number?
Show answer
117
TRY IT 2.2
![]() of
of ![]() is what number?
is what number?
Show answer
126
In the next examples, we are asked to find the base.
EXAMPLE 3
Translate and solve: ![]() is
is ![]() of what number?
of what number?
| Translate. Let |
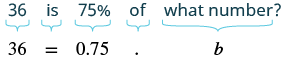 |
| Divide both sides by 0.75. |  |
| Simplify. | 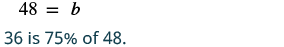 |
TRY IT 3.1
![]() is
is ![]() of what number?
of what number?
Show answer
68
TRY IT 3.2
![]() is
is ![]() of what number?
of what number?
Show answer
64
EXAMPLE 4
![]() of what number is
of what number is ![]()
| Translate. Let |
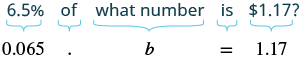 |
| Divide both sides by 0.065. | 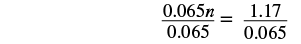 |
| Simplify. |  |
TRY IT 4.1
![]() of what number is
of what number is ![]()
Show answer
$26
TRY IT 4.1
![]() of what number is
of what number is ![]()
Show answer
$36
In the next examples, we will solve for the percent.
EXAMPLE 5
What percent of ![]() is
is ![]()
| Translate into algebra. Let |
 |
| Divide by 36. |  |
| Simplify. |  |
| Convert to decimal form. |  |
| Convert to percent. | 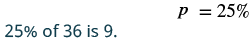 |
TRY IT 5.1
What percent of ![]() is
is ![]()
Show answer
75%
TRY IT 5.2
What percent of ![]() is
is ![]()
Show answer
80%
EXAMPLE 6
![]() is what percent of
is what percent of ![]()
| Translate into algebra. Let |
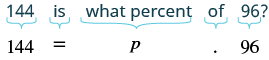 |
| Divide by 96. |  |
| Simplify. |  |
| Convert to percent. |  |
TRY IT 6.1
![]() is what percent of
is what percent of ![]()
Show answer
125%
TRY IT 6.2
![]() is what percent of
is what percent of ![]()
Show answer
175%
Solve Applications of Percent
Many applications of percent occur in our daily lives, such as tips, sales tax, discount, and interest. To solve these applications we’ll translate to a basic percent equation, just like those we solved in the previous examples in this section. Once you translate the sentence into a percent equation, you know how to solve it.
We will update the strategy we used in our earlier applications to include equations now. Notice that we will translate a sentence into an equation.
HOW TO: Solve an Application
- Identify what you are asked to find and choose a variable to represent it.
- Write a sentence that gives the information to find it.
- Translate the sentence into an equation.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Write a complete sentence that answers the question.
Now that we have the strategy to refer to, and have practiced solving basic percent equations, we are ready to solve percent applications. Be sure to ask yourself if your final answer makes sense—since many of the applications we’ll solve involve everyday situations, you can rely on your own experience.
EXAMPLE 7
Dezohn and his girlfriend enjoyed a dinner at a restaurant, and the bill was ![]() . They want to leave an
. They want to leave an ![]() tip. If the tip will be
tip. If the tip will be ![]() of the total bill, how much should the tip be?
of the total bill, how much should the tip be?
| What are you asked to find? | The amount of the tip |
| Choose a variable to represent it. | Let |
| Write a sentence that give the information to find it. | The tip is 18% of the total bill. |
| Translate the sentence into an equation. |  |
| Multiply. | 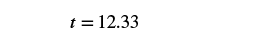 |
| Check. Is this answer reasonable? | |
| If we approximate the bill to $70 and the percent to 20%, we would have a tip of $14. So a tip of $12.33 seems reasonable. |
|
| Write a complete sentence that answers the question. | The couple should leave a tip of $12.33. |
TRY IT 7.1
Cierra and her sister enjoyed a special dinner in a restaurant, and the bill was ![]() . If she wants to leave
. If she wants to leave ![]() of the total bill as her tip, how much should she leave?
of the total bill as her tip, how much should she leave?
Show answer
$14.67
TRY IT 7.2
Kimngoc had lunch at her favorite restaurant. She wants to leave ![]() of the total bill as her tip. If her bill was
of the total bill as her tip. If her bill was ![]() , how much will she leave for the tip?
, how much will she leave for the tip?
Show answer
$2.16
EXAMPLE 8
The label on Masao’s breakfast cereal said that one serving of cereal provides ![]() milligrams (mg) of potassium, which is
milligrams (mg) of potassium, which is ![]() of the recommended daily amount. What is the total recommended daily amount of potassium?
of the recommended daily amount. What is the total recommended daily amount of potassium?
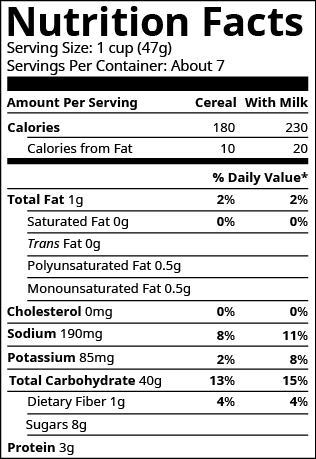
| What are you asked to find? | the total amount of potassium recommended |
| Choose a variable to represent it. | Let |
| Write a sentence that gives the information to find it. | 85 mg is 2% of the total amount. |
| Translate the sentence into an equation. |  |
| Divide both sides by 0.02. | 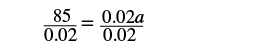 |
| Simplify. |  |
| Check: Is this answer reasonable? | |
| Yes. 2% is a small percent and 85 is a small part of 4,250. | |
| Write a complete sentence that answers the question. | The amount of potassium that is recommended is 4250 mg. |
TRY IT 8.1
One serving of wheat square cereal has ![]() grams of fiber, which is
grams of fiber, which is ![]() of the recommended daily amount. What is the total recommended daily amount of fiber?
of the recommended daily amount. What is the total recommended daily amount of fiber?
Show answer
24.1 grams
TRY IT 8.2
One serving of rice cereal has ![]() mg of sodium, which is
mg of sodium, which is ![]() of the recommended daily amount. What is the total recommended daily amount of sodium?
of the recommended daily amount. What is the total recommended daily amount of sodium?
Show answer
2,375 mg
EXAMPLE 9
Mitzi received some gourmet brownies as a gift. The wrapper said each brownie was ![]() calories, and had
calories, and had ![]() calories of fat. What percent of the total calories in each brownie comes from fat?
calories of fat. What percent of the total calories in each brownie comes from fat?
| What are you asked to find? | the percent of the total calories from fat |
| Choose a variable to represent it. | Let |
| Write a sentence that gives the information to find it. | What percent of 480 is 240? |
| Translate the sentence into an equation. |  |
| Divide both sides by 480. | 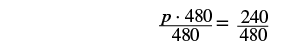 |
| Simplify. |  |
| Convert to percent form. |  |
| Check. Is this answer reasonable? | |
| Yes. 240 is half of 480, so 50% makes sense. | |
| Write a complete sentence that answers the question. | Of the total calories in each brownie, 50% is fat. |
TRY IT 9.1
Veronica is planning to make muffins from a mix. The package says each muffin will be ![]() calories and
calories and ![]() calories will be from fat. What percent of the total calories is from fat? (Round to the nearest whole percent.)
calories will be from fat. What percent of the total calories is from fat? (Round to the nearest whole percent.)
Show answer
26%
Exercises
The brownie mix Ricardo plans to use says that each brownie will be ![]() calories, and
calories, and ![]() calories are from fat. What percent of the total calories are from fat?
calories are from fat. What percent of the total calories are from fat?
Show answer
37%
Find Percent Increase and Percent Decrease
People in the media often talk about how much an amount has increased or decreased over a certain period of time. They usually express this increase or decrease as a percent.
To find the percent increase, first we find the amount of increase, which is the difference between the new amount and the original amount. Then we find what percent the amount of increase is of the original amount.
HOW TO: Find Percent Increase
Step 1. Find the amount of increase.
Step 2. Find the percent increase as a percent of the original amount.
EXAMPLE 10
In ![]() , university tuition fees in Canada for domestic students increased from
, university tuition fees in Canada for domestic students increased from ![]() per school year to
per school year to ![]() per school year. Find the percent increase. (Round to the nearest tenth of a percent.)
per school year. Find the percent increase. (Round to the nearest tenth of a percent.)
| What are you asked to find? | the percent increase |
| Choose a variable to represent it. | Let |
| Find the amount of increase. | 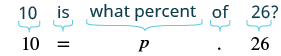 |
| Find the percent increase. | The increase is what percent of the original amount? |
| Translate to an equation. | |
| Divide both sides by 26. |  |
| Round to the nearest thousandth. |  |
| Convert to percent form. |  |
| Write a complete sentence. | The new fees represent a 38.4\% increase over the old fees. |
TRY IT 10.1
In ![]() , the IRS increased the deductible mileage cost to
, the IRS increased the deductible mileage cost to ![]() cents from
cents from ![]() cents. Find the percent increase. (Round to the nearest tenth of a percent.)
cents. Find the percent increase. (Round to the nearest tenth of a percent.)
Show answer
8.8%
TRY IT 10.2
In ![]() , the standard bus fare in Vancouver was
, the standard bus fare in Vancouver was ![]() . In
. In ![]() , the standard bus fare was
, the standard bus fare was ![]() . Find the percent increase. (Round to the nearest tenth of a percent.)
. Find the percent increase. (Round to the nearest tenth of a percent.)
Show answer
50%
Finding the percent decrease is very similar to finding the percent increase, but now the amount of decrease is the difference between the original amount and the final amount. Then we find what percent the amount of decrease is of the original amount.
HOW TO: Find Percent Decrease
- Find the amount of decrease.
- Find the percent decrease as a percent of the original amount.
EXAMPLE 11
The average price of a gallon of gas in one city in June ![]() was
was ![]() . The average price in that city in July was
. The average price in that city in July was ![]() . Find the percent decrease.
. Find the percent decrease.
| What are you asked to find? | the percent decrease |
| Choose a variable to represent it. | Let |
| Find the amount of decrease. |  |
| Find the percent of decrease. | The decrease is what percent of the original amount? |
| Translate to an equation. | 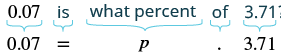 |
| Divide both sides by 3.71. |  |
| Round to the nearest thousandth. |  |
| Convert to percent form. |  |
| Write a complete sentence. | The price of gas decreased 1.9%. |
TRY IT 11.1
The population of one city was about ![]() in
in ![]() . The population of the city is projected to be about
. The population of the city is projected to be about ![]() in
in ![]() . Find the percent decrease. (Round to the nearest tenth of a percent.)
. Find the percent decrease. (Round to the nearest tenth of a percent.)
Show answer
6.3%
TRY IT 11.2
Last year Sheila’s salary was ![]() . Because of furlough days, this year her salary was
. Because of furlough days, this year her salary was ![]() . Find the percent decrease. (Round to the nearest tenth of a percent.)
. Find the percent decrease. (Round to the nearest tenth of a percent.)
Show answer
10%
Access Additional Online Resources
Key Concepts
- Solve an application.
- Identify what you are asked to find and choose a variable to represent it.
- Write a sentence that gives the information to find it.
- Translate the sentence into an equation.
- Solve the equation using good algebra techniques.
- Write a complete sentence that answers the question.
- Check the answer in the problem and make sure it makes sense.
- Find percent increase.
- Find the amount of increase:
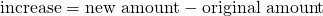
- Find the percent increase as a percent of the original amount.
- Find the amount of increase:
- Find percent decrease.
- Find the amount of decrease.

- Find the percent decrease as a percent of the original amount.
- Find the amount of decrease.
Glossary
- percent increase
- The percent increase is the percent the amount of increase is of the original amount.
- percent decrease
- The percent decrease is the percent the amount of decrease is of the original amount.
Practice Makes Perfect
Translate and Solve Basic Percent Equations
In the following exercises, translate and solve.
| 1. What number is |
2. What number is |
| 3. What number is |
4. What number is |
| 5. |
6. |
| 7. |
8. |
| 9. |
10. |
| 11. |
12. |
| 13. |
14. |
| 15. |
16. |
| 17. What percent of |
18. What percent of |
| 19. What percent of |
20. What percent of |
| 21. |
22. |
| 23. |
24. |
Solve Applications of Percents
In the following exercises, solve the applications of percents.
| 25. Geneva treated her parents to dinner at their favorite restaurant. The bill was |
26. When Hiro and his co-workers had lunch at a restaurant the bill was |
| 27. Trong has |
28. Cherise deposits |
| 29. One serving of oatmeal has |
30. One serving of trail mix has |
| 31. A bacon cheeseburger at a popular fast food restaurant contains |
32. A grilled chicken salad at a popular fast food restaurant contains |
| 33. The nutrition fact sheet at a fast food restaurant says the fish sandwich has |
34. The nutrition fact sheet at a fast food restaurant says a small portion of chicken nuggets has |
| 35. Emma gets paid |
36. Dimple gets paid |
Find Percent Increase and Percent Decrease
In the following exercises, find the percent increase or percent decrease.
| 37. Tamanika got a raise in her hourly pay, from |
38. Ayodele got a raise in her hourly pay, from |
| 39. According to Statistics Canada, annual international graduate student fees in Canada rose from about |
40. The price of a share of one stock rose from |
| 41. According to Time magazine |
42. In one month, the median home price in the Northeast rose from |
| 43. A grocery store reduced the price of a loaf of bread from |
44. The price of a share of one stock fell from |
| 45. Hernando’s salary was |
46. From |
| 47. In one month, the median home price in the West fell from |
48. Sales of video games and consoles fell from |
Everyday Math
| 49. Tipping At the campus coffee cart, a medium coffee costs |
50. Late Fees Alison was late paying her credit card bill of |
Writing Exercises
| 51. Without solving the problem |
52. Without solving the problem “What is |
| 53. After returning from vacation, Alex said he should have packed |
54. Because of road construction in one city, commuters were advised to plan their Monday morning commute to take |
Answers
| 1. 54 | 3. 26.88 | 5. 162.5 |
| 7. 18,000 | 9. 112 | 11. 108 |
| 13. $35 | 15. $940 | 17. 30% |
| 19. 36% | 21. 150% | 23. 175% |
| 25. $11.88 | 27. $259.80 | 29. 24.2 grams |
| 31. 2,407 grams | 33. 45% | 35. 25% |
| 37. 13.2% | 39. 15% | 41. 72.7% |
| 43. 2.5% | 45. 11% | 47. 5.5% |
| 49. 21.2% | 51. The original number should be greater than 44.80% is less than 100%, so when 80% is converted to a decimal and multiplied to the base in the percent equation, the resulting amount of 44 is less. 44 is only the larger number in cases where the percent is greater than 100%. | 53. Alex should have packed half as many shorts and twice as many shirts. |
Attributions
This chapter has been adapted from “Solve General Applications of Percent” in Prealgebra (OpenStax) by Lynn Marecek, MaryAnne Anthony-Smith, and Andrea Honeycutt Mathis, which is under a CC BY 4.0 Licence. Adapted by Izabela Mazur. See the Copyright page for more information.

